Full solution
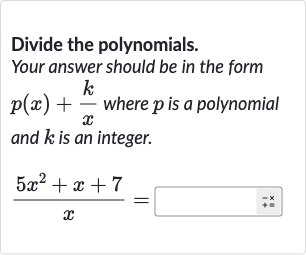
Q. Divide the polynomials. Your answer should be in the form where is a polynomial and is an integer.
- Step : Divide by x: Divide each term of the polynomial by x.We will divide each term of the polynomial by separately. divided by is , because in the denominator cancels out one in the numerator. divided by is , because is equal to . divided by cannot be simplified further and remains as .
- Step : Write in form: Write the result in the form .The result of the division from Step gives us the polynomial part as and the fraction part as .So, the final answer in the required form is .