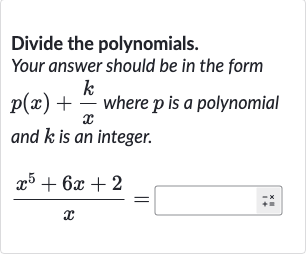
Divide the polynomials. Your answer should be in the form p(x)+(k)/(x) where p is a polynomial and k is an integer. (x^(5)+6x+2)/(x)=◻

Question

Divide the polynomials. Your answer should be in the form  p(x)+(k)/(x) where  p is a polynomial and  k is an integer.  (x^(5)+6x+2)/(x)=◻

Bytelearn · Accepted Answer

Divide by x: Divide each term of the polynomial by x. We will divide each term of the polynomial x^5 + 6x + 2 by x separately.Divide x^5 by x: Divide the first term x^5 by x. x^5 divided by x is x^4 because when dividing powers with the same base, we subtract the exponents. Calculation: x^5 / x = x^(5-1) = x^4Divide 6x by x: Divide the second term 6x by x. 6x divided by x is 6 because the x terms cancel out. Calculation: 6x / x = 6Divide 2 by x: Divide the third term 2 by x. 2 divided by x cannot be simplified further and will remain as the fraction 2/x. Calculation: 2 / x = 2/xCombine the results: Combine the results from steps 2, 3, and 4. The combined result of the division is the polynomial part plus the fraction. Calculation: x^4 + 6 + 2/xWrite the final answer: Write the final answer in the form p(x) + (k)/(x). The polynomial part is p(x) = x^4 + 6, and the fraction part is (k)/(x) = 2/x. Final Answer: x^4 + 6 + 2/x

Divide the polynomials. $\newline$ Your answer should be in the form $p(x)+\frac{k}{x}$ where $p$ is a polynomial and $k$ is an integer. $\newline$ $\frac{x^{5}+6 x+2}{x}=\square$

Full solution

More problems from Powers with decimal and fractional bases

Related topics

Divide the polynomials.\newlineYour answer should be in the form p(x)+kx p(x)+\frac{k}{x} p(x)+xk​ where p p p is a polynomial and k k k is an integer.\newlinex5+6x+2x=□ \frac{x^{5}+6 x+2}{x}=\square xx5+6x+2​=□

Divide the polynomials. $\newline$ Your answer should be in the form $p(x)+\frac{k}{x}$ where $p$ is a polynomial and $k$ is an integer. $\newline$ $\frac{x^{5}+6 x+2}{x}=\square$