Full solution
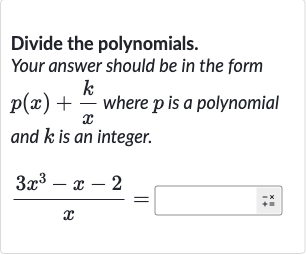
Q. Divide the polynomials.Your answer should be in the form where is a polynomial and is an integer.
- Identify dividend and divisor: Identify the dividend and the divisor. The dividend is the polynomial , and the divisor is .
- Divide each term by x: Divide each term of the polynomial by x.To divide a polynomial by x, divide each term of the polynomial by x individually.
- Simplify each term: Simplify each term after division. (since ) (since any non-zero number divided by itself is ) remains as it is because is not divisible by .So,
- Write final answer: Write the final answer in the required form.The polynomial part is , and the integer is .Therefore, the final answer is .