A circle in the $x y$ -plane has the equation $\newline$ $(x+17.5)^{2}+(y-15 . \overline{3})^{2}=18.1$ $\newline$ Which of the following best describes the location of the center of the circle and the length of its radius? $\newline$ Choose $1$ answer: $\newline$ (A) Center: $(-17.5,15 . \overline{3})$ $\newline$ Radius: $\sqrt{18.1}$ $\newline$ (B) Center: $(-17.5,15 . \overline{3})$ $\newline$ Radius: $18$ . $1$ $\newline$ (C) Center: $(17.5,-15 . \overline{3})$ $\newline$ Radius: $\sqrt{18.1}$ $\newline$ (D) Center: $(17.5,-15 . \overline{3})$ $\newline$ Radius: $18$ . $1$

Full solution

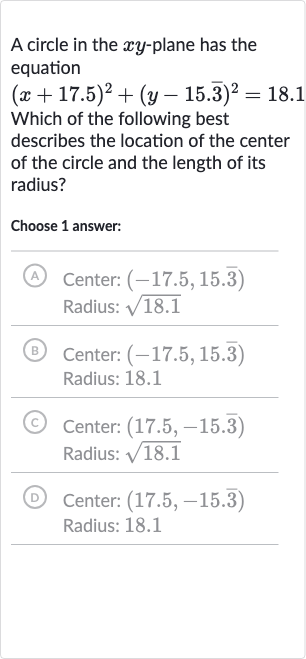
Q. A circle in the

x y

-plane has the equation

\newline

(x+17.5)^{2}+(y-15 . \overline{3})^{2}=18.1

\newline

Which of the following best describes the location of the center of the circle and the length of its radius?

\newline

Choose

1

answer:

\newline

(A) Center:

(-17.5,15 . \overline{3})

\newline

Radius:

\sqrt{18.1}

\newline

(B) Center:

(-17.5,15 . \overline{3})

\newline

Radius:

18

1

\newline

(17.5,-15 . \overline{3})

\newline

Radius:

\sqrt{18.1}

\newline

(D) Center:

(17.5,-15 . \overline{3})

\newline

Radius:

18

1

Identifying the Center: The equation of a circle in the xy-plane is given by the formula $(x - h)^2 + (y - k)^2 = r^2$ , where $(h, k)$ is the center of the circle and $r$ is the radius. We need to compare this formula with the given equation $(x+17.5)^2 + (y-15.\overline{3})^2 = 18.1$ to find the center and the radius of the circle.
Determining the Radius: First, we identify the center of the circle by looking at the terms $(x+17.5)^2$ and $(y-15.\overline{3})^2$ . According to the standard form of the circle's equation, the center $(h, k)$ will be the opposite signs of the values inside the parentheses. Therefore, the center is $(-17.5, 15.\overline{3})$ .
Comparing with Answer Choices: Next, we determine the radius of the circle. The radius squared $r^2$ is equal to the constant term on the right side of the equation, which is $18.1$ . To find the radius $r$ , we take the square root of $18.1$ , which is $\sqrt{18.1}$ .
Comparing with Answer Choices: Next, we determine the radius of the circle. The radius squared $r^2$ is equal to the constant term on the right side of the equation, which is $18.1$ . To find the radius $r$ , we take the square root of $18.1$ , which is $\sqrt{18.1}$ .Now we can compare our findings with the given answer choices. The center we found is $(-17.5, 15.\overline{3})$ and the radius is $\sqrt{18.1}$ . This matches with option (A).