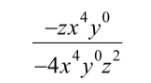Resourcesdown chevron
Testimonials
Plans
Sign in
Sign up
Resourcesright arrow
Testimonials
Plans
Bytelearn - cat image with glassesAI tutor

Welcome to Bytelearn!

Let’s check out your problem:

(-zx^(4)y^(0))/(-4x^(4)y^(0)z^(2))

−zx4y0−4x4y0z2 \frac{-z x^{4} y^{0}}{-4 x^{4} y^{0} z^{2}} =

View step-by-step helpView step-by-step help
Homeright chevron icon
Math Problemsright chevron icon
Calculusright chevron icon
Find derivatives of using multiple formulaeright chevron icon

Full solution

Q. −zx4y0−4x4y0z2 \frac{-z x^{4} y^{0}}{-4 x^{4} y^{0} z^{2}} =
  1. Simplify powers of y: First, simplify the powers of y since y0=1y^0 = 1.−zx4⋅1−4x4⋅1⋅z2=−zx4−4x4z2\frac{-zx^4 \cdot 1}{-4x^4 \cdot 1 \cdot z^2} = \frac{-zx^4}{-4x^4z^2}
  2. Cancel out common terms: Next, simplify the expression by canceling out common terms. The x4x^4 terms cancel out and one zz in the numerator and denominator cancels out. −z4z=−14\frac{-z}{4z} = -\frac{1}{4}

More problems from Find derivatives of using multiple formulae

Question
Find the derivative of f(x) f(x) .\newlinef(x)=cos⁡(x) f(x) = \cos(x) \newlinef′(x)= f'(x) = ______
Get tutor helpright-arrow

Posted 1 month ago

Question
Find the derivative of f(x) f(x) .\newlinef(x)=tan⁡(x) f(x) = \tan(x) \newlinef′(x)= f'(x) = ______
Get tutor helpright-arrow

Posted 2 months ago

Question
Find the derivative of f(x) f(x) .\newlinef(x)=ex f(x) = e^x \newlinef′(x)= f'\left(x\right) = ______
Get tutor helpright-arrow

Posted 2 months ago

Question
Find the derivative of f(x) f(x) .\newlinef(x)=ln⁡(x) f(x) = \ln(x) \newlinef′(x)= f'(x) = ______
Get tutor helpright-arrow

Posted 2 months ago

Question
Find the derivative of f(x) f(x) .\newlinef(x)=tan⁡−1x f(x) = \tan^{-1}{x} \newlinef′(x)= f'(x) = ______
Get tutor helpright-arrow

Posted 2 months ago

Question
Find the derivative of f(x) f(x) .\newlinef(x)=xex f(x) = x e^x \newlinef′(x)= f'\left(x\right) = ______
Get tutor helpright-arrow

Posted 2 months ago

Question
Find the derivative of f(x) f(x) . \newlinef(x)=exx f(x) = \frac{e^x}{x} \newlinef′(x)= f'(x) = ______
Get tutor helpright-arrow

Posted 2 months ago

Question
Find the derivative of f(x) f(x) . \newline f(x)=e(x+1) f(x) = e^{(x + 1)} \newline f′(x)= f'\left(x\right) = ______
Get tutor helpright-arrow

Posted 1 month ago

Question
Find the derivative of f(x) f(x) . \newlinef(x)=x+3 f(x) = \sqrt{x+3} \newlinef′(x)= f'(x) = ______
Get tutor helpright-arrow

Posted 2 months ago

Question
Find the derivative of g(x) g(x). \newline g(x)=ln⁡(2x) g(x) = \ln(2x) \newline g′(x)= g^{\prime}(x) = ______
Get tutor helpright-arrow

Posted 3 months ago

View all (665)