Full solution
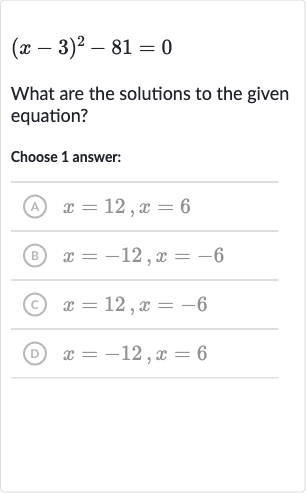
Q. What are the solutions to the given equation?Choose answer:(A) (B) (C) (D)
- Setting the equation and identifying terms: First, we need to set the equation equal to zero and identify the terms.This is a quadratic equation in the form of a difference of squares.
- Applying the difference of squares formula: Next, we apply the difference of squares formula, which is , where and .
- Simplifying the factors: Now, we simplify the factors.So, the equation becomes:
- Setting each factor equal to zero: To find the solutions, we set each factor equal to zero. or
- Solving for x in the first equation: Solve for in the first equation.
- Solving for x in the second equation: Solve for x in the second equation.
More problems from Solve linear equations: mixed review
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help