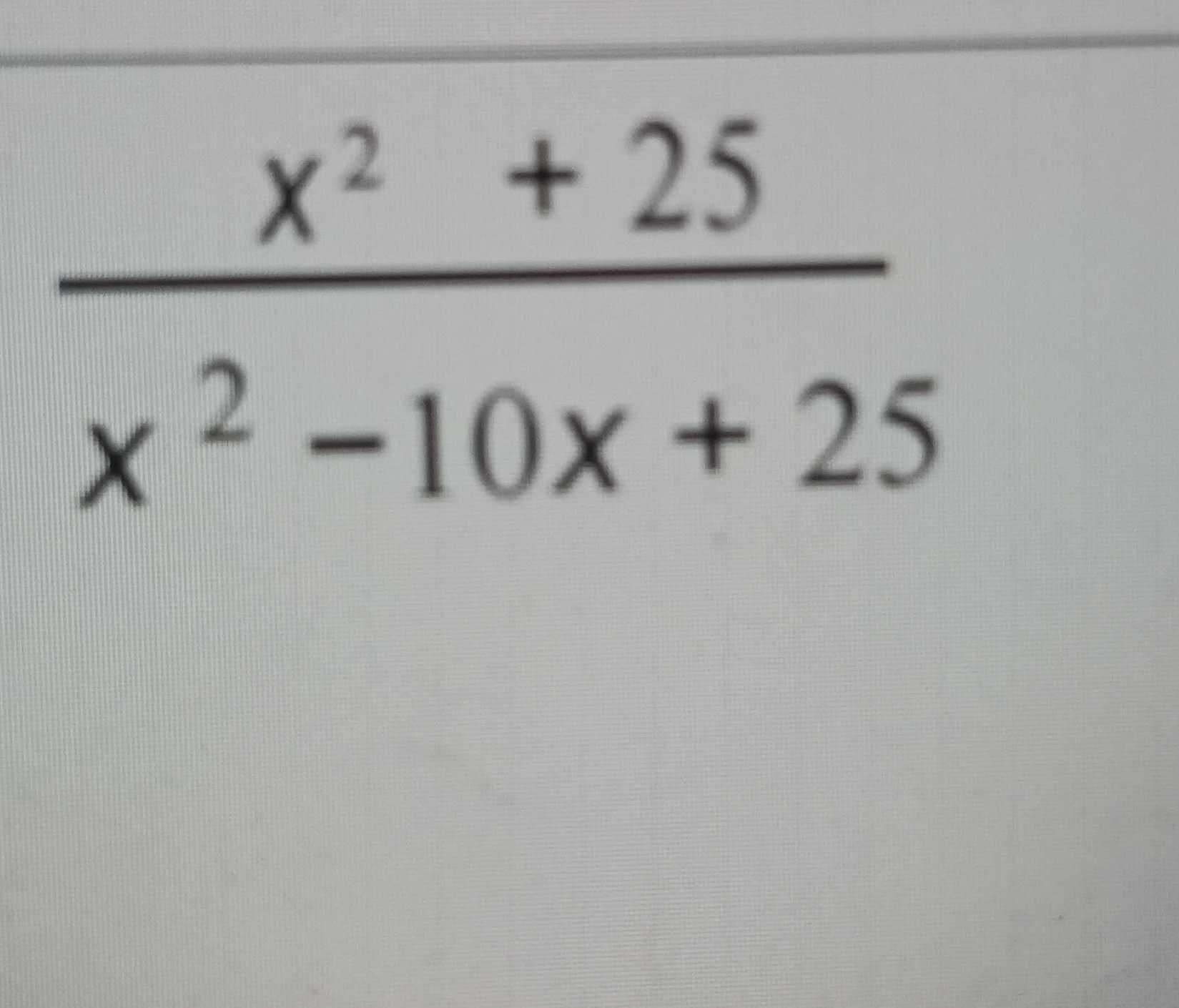AI tutor
Full solution
Q. Simplify the expression:
- Identify function: Identify the function to differentiate.Function:
- Apply quotient rule: Apply the quotient rule: . Let and . Then, and .
- Substitute into formula: Substitute into the quotient rule formula.
- Expand and simplify: Expand and simplify the numerator.Numerator = - = (-10\)x^ + x +