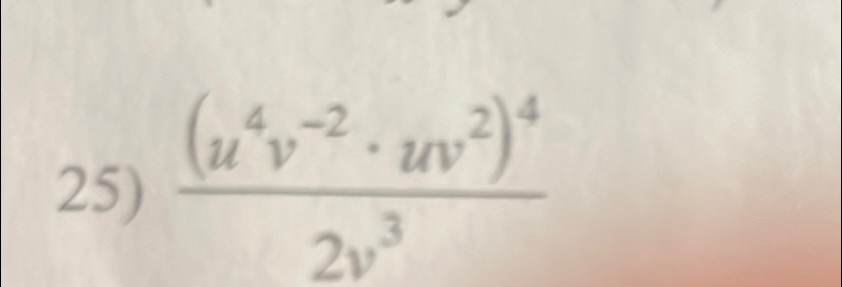AI tutor
Full solution
Q. )
- Simplify Inside Parentheses: First, simplify the expression inside the parentheses before raising it to the fourth power., since any number to the power of is .
- Raise to Fourth Power: Now raise the simplified expression inside the parentheses to the fourth power. .
- Divide by Denominator: Next, divide the result by the denominator .
- Final Simplified Form: Finally, write the expression in its simplified form. is already in its simplest form.