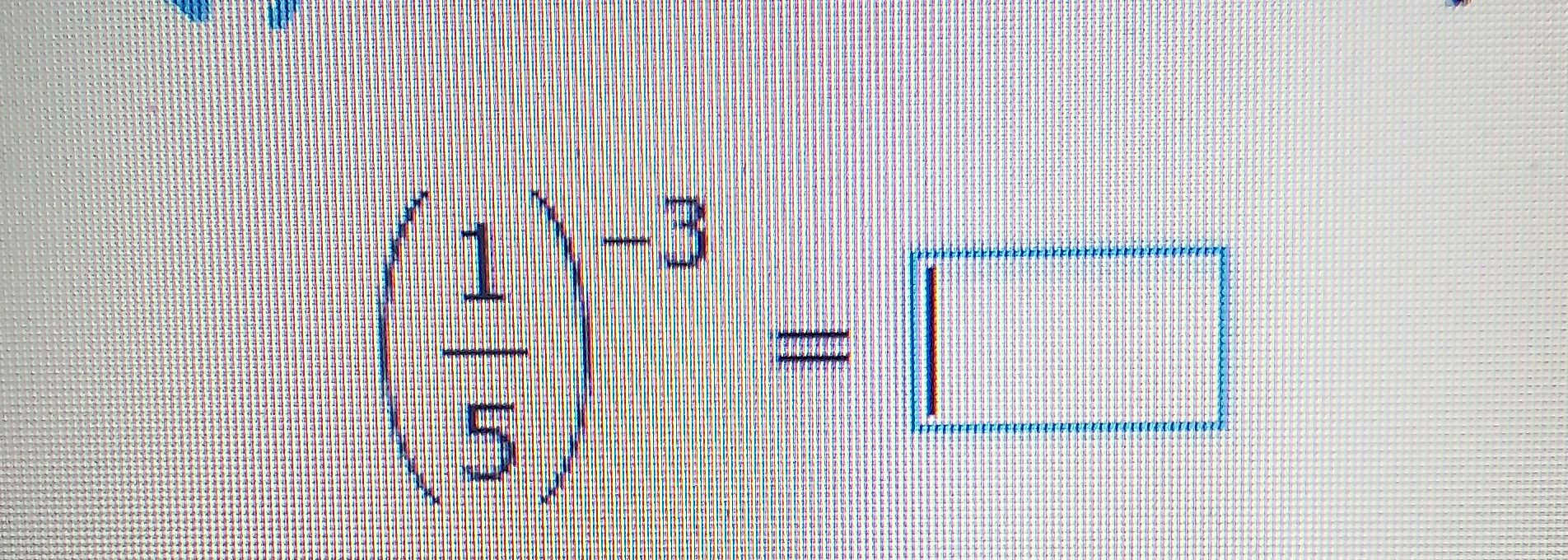Resources

Resources

Bytelearn - cat image with glasses

AI tutor

Welcome to Bytelearn!

Let’s check out your problem:

((1)/(5))^(-3)=

$\left(\frac{1}{5}\right)^{-3}=$

View step-by-step help

View step-by-step help

Powers with negative bases

Full solution

Q.

\left(\frac{1}{5}\right)^{-3}=

Identify base and exponent: Identify the base and the exponent. $\newline$ In the expression $\left(\frac{1}{5}\right)^{-3}$ , $\left(\frac{1}{5}\right)$ is the base raised to the exponent $-3$ . $\newline$ Base: $\frac{1}{5}$ $\newline$ Exponent: $-3$
Understand negative exponent rule: Understand the negative exponent rule. A negative exponent means that we take the reciprocal of the base and change the sign of the exponent to positive. So, $(\frac{1}{5})^{-3}$ means we take the reciprocal of $\frac{1}{5}$ and raise it to the power of $3$ . Reciprocal of $\frac{1}{5}$ : $\frac{5}{1}$ or simply $5$
Apply negative exponent rule: Apply the negative exponent rule. $\newline$ $(\frac{1}{5})^{-3}$ becomes $(\frac{5}{1})^3$ .
Calculate value of $(5/1)^3$ : Calculate the value of $(5/1)^3$ . $(5/1)^3$ means $5$ is multiplied by itself $3$ times. $(5/1)^3 = 5 \times 5 \times 5$
Perform multiplication: Perform the multiplication. $5 \times 5 \times 5 = 125$
Write final answer: Write the final answer. $\newline$ The value of $\left(\frac{1}{5}\right)^{-3}$ is $125$ .

More problems from Powers with negative bases

Question

Write the expression using an exponent.

\newline

2 \times 2

\newline

Posted 3 months ago

Question

Evaluate the expression.

4 - 4^2

Posted 3 months ago

Question

x^2 = 4

Posted 2 months ago

Question

x^2 = 9

Posted 4 months ago

Question

\newline

x^2 = 0

\newline

Write your answer in simplified, rationalized form.

\newline

x =

x =

Posted 4 months ago

Question

x

\newline

8 = 2^x

\newline

x =

Posted 4 months ago

Question

x

\newline

6 = 7^x

\newline

x =

Posted 4 months ago

Question

Select the equivalent expression.

\newline

((8^{-5})/(2^{-2}))^{-4}=?

\newline

1

\newline

\newline

(8^{20})/(2^{8})

\newline

\newline

(2^{6})/(8^{9})

\newline

\newline

(1)/(8\cdot 2^{2})

Posted 17 days ago

Question

Select the equivalent expression.

\newline

\left(\frac{b^{7}}{4^{5}}\right)^{-3}=\,?

\newline

1

\newline

\frac{b^{21}}{4^{15}}

\newline

\frac{4^{15}}{b^{21}}

\newline

b^{-21}\cdot 4^{-15}

Posted 2 months ago

Question

Select the equivalent expression.

\newline

(3^{3}\cdot6^{6})^{-3}=

\newline

1

\newline

\frac{1}{3^{9}\cdot6^{18}}

\newline

\frac{6^{18}}{3^{9}}

\newline

\frac{3^{9}}{6^{18}}

Posted 2 months ago