Resources

Resources

Bytelearn - cat image with glasses

AI tutor

Welcome to Bytelearn!

Let’s check out your problem:

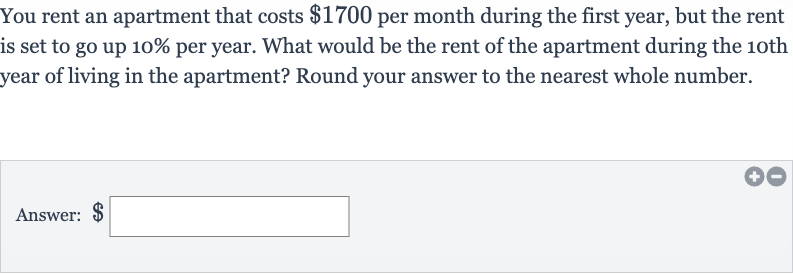
You rent an apartment that costs
$1700 per month during the first year, but the rent is set to go up 10% per year. What would be the rent of the apartment during the 10th year of living in the apartment? Round your answer to the nearest whole number.
Answer:
$ □

You rent an apartment that costs $\$ 1700$ per month during the first year, but the rent is set to go up $10$ \% per year. What would be the rent of the apartment during the $10$ th year of living in the apartment? Round your answer to the nearest whole number. $\newline$ Answer: $\$$ $\square$

View step-by-step help

View step-by-step help

Exponential growth and decay: word problems

Full solution

Q. You rent an apartment that costs

\$ 1700

per month during the first year, but the rent is set to go up

10

\% per year. What would be the rent of the apartment during the

10

th year of living in the apartment? Round your answer to the nearest whole number.

\newline

Answer:

\$

\square

Identify rent details: Identify the initial rent and the annual percentage increase. $\newline$ The initial rent is $\$1700$ per month, and the rent increases by $10\%$ each year.
Determine formula for calculation: Determine the formula for calculating the rent after a certain number of years. $\newline$ The rent after $n$ years can be calculated using the formula for exponential growth: $\text{Rent}_n = \text{Rent}_0 \times (1 + \text{rate})^n$ , where $\text{Rent}_0$ is the initial rent, $\text{rate}$ is the annual increase rate (as a decimal), and $n$ is the number of years.
Convert percentage to decimal: Convert the percentage increase to a decimal. $\newline$ A $10\%$ increase is equivalent to $0.10$ in decimal form.
Calculate rent for $10$ th year: Calculate the rent for the $10$ th year using the formula. $\text{Rent}_{10} = 1700 \times (1 + 0.10)^{10}$
Perform calculation: Perform the calculation. $\newline$ $Rent_{10} = 1700 \times (1.10)^{10}$ $\newline$ $Rent_{10} = 1700 \times 2.5937424601$ (using a calculator for $1.10^{10}$ ) $\newline$ $Rent_{10} = 4409.36158217$
Round answer: Round the answer to the nearest whole number. $\text{Rent}_{10} \approx \$(4409)$

More problems from Exponential growth and decay: word problems

Question

Use the following function rule to find

f(2)

\newline

f(x) = 11 \cdot (9)^x + 8

\newline

f(2) =

Posted 5 months ago

Question

Raymond works for a pharmaceutical company that is testing the effectiveness of a new medication. He gave one of the study participants

400

milligrams of the medication, and he knows the amount remaining in the participant's body will decrease by a factor of

1/3

\newline

Write an exponential equation in the form

y = a(b)^x

that can model the amount of medication,

y

, remaining in the participant's body after

x

\newline

Use whole numbers, decimals, or simplified fractions for the values of

a

b

\newline

y =

\newline

Posted 8 months ago

Question

100

bacteria doubles in size every

60

hours. What will the population be

180

?

Posted 4 months ago

Question

Charlotte opened a savings account and deposited

\$800.00

as principal. The account earns

8\%

interest, compounded annually. What is the balance after

10

\newline

Use the formula

A = P(1 + \frac{r}{n})^{nt}

A

is the balance (final amount),

P

is the principal (starting amount),

r

is the interest rate expressed as a decimal,

n

is the number of times per year that the interest is compounded, and

t

is the time in years.

\newline

Round your answer to the nearest cent.

Posted 4 months ago

Question

Bob rented a car for a flat fee of `$150` and an additional `$10` per day. Write a linear function that represents the total cost,

f(x)

x

Posted 10 months ago

Question

Sally purchased a laptop for `$800` and has to pay a service fee of `$20` for each day until it is delivered. Write a linear function to represent the total cost,

h(x)

x

Posted 10 months ago

Question

\$1,000

in a savings account with a daily interest rate of

0.1\%

. Write an exponential function to represent the total amount,

s(x)

, in his account after

x

Posted 9 months ago

Question

A=2450+740 s

\newline

The total amount,

A

, in thousands of dollars, of scholarship money given out by a college throughout its history at a time of

s

semesters after the summer of the year

2000

is given by the equation. What was the total amount of scholarship money, in thousands of dollars, given out by the college up to the summer of the year

2000

Posted 5 months ago

Question

T=235+980 d

\newline

T

, in newton-meters, on a diving board with a

100

kilogram weight placed

d

meters from the diving board's fulcrum is given by the equation. How much torque in newton-meters is on the diving board when the weight is placed at the fulcrum?

Posted 8 months ago

Question

R=42-0.7 t

\newline

Quinn returned home one summer's day to find it extremely hot. He turned the air conditioner on, and the room's temperature began decreasing at a constant rate. The given equation shown gives the room's temperature,

R

, in degrees Celsius,

t

minutes after Quinn turned on the air conditioner. What was the room's temperature, in degrees Celsius, when Quinn returned home?

Posted 7 months ago

Related topics

Algebra - Order of Operations Algebra - Distributive Property `X` and `Y` Axes Geometry - Scalene Triangle Common Multiple Geometry - Quadrant