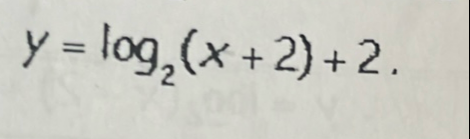AI tutor
Full solution
Q.
- Understand given logarithmic equation: Understand the given logarithmic equation.We are given the equation , where denotes the logarithm base . We need to solve for .
- Isolate logarithmic term: Isolate the logarithmic term.To solve for , we first need to isolate the logarithmic term. We can do this by subtracting from both sides of the equation.
- Convert to exponential equation: Convert the logarithmic equation to an exponential equation.The inverse of a logarithm is an exponentiation. We can rewrite the equation in exponential form using the definition of a logarithm: if , then .
- Isolate x: Isolate . Now we need to isolate by subtracting from both sides of the equation.