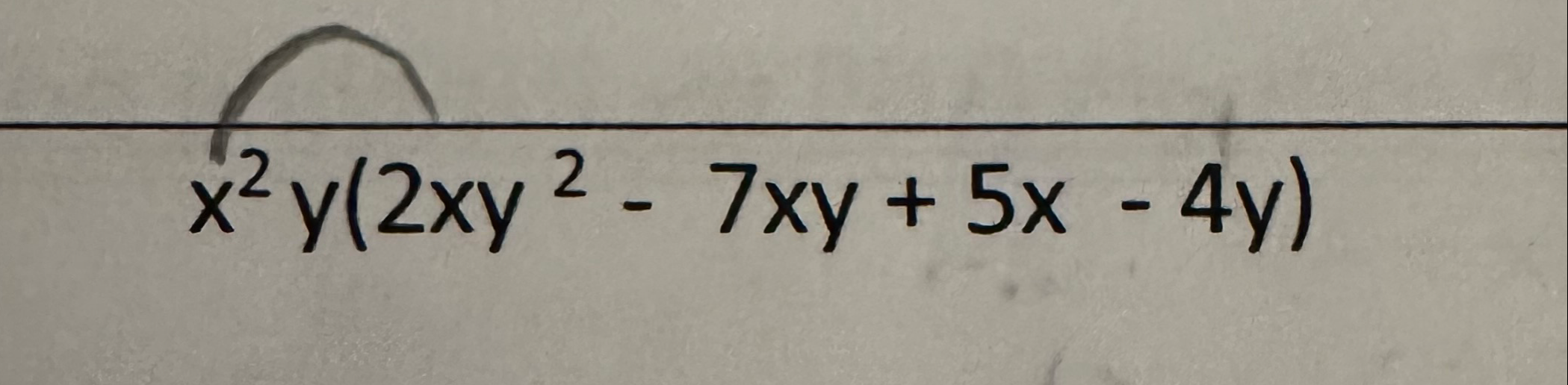AI tutor
Full solution
Q.
- Distribute : Distribute across the terms inside the parentheses . To distribute, multiply by each term inside the parentheses.
- Multiply : Multiply by the first term .
- Multiply : Multiply by the second term .
- Multiply : Multiply by the third term .
- Multiply : Multiply by the fourth term .
- Combine results: Combine the results from steps to to get the final expression.
More problems from Quadratic equation with complex roots
QuestionGet tutor help
QuestionGet tutor help