Resources

Resources

Bytelearn - cat image with glasses

AI tutor

Welcome to Bytelearn!

Let’s check out your problem:

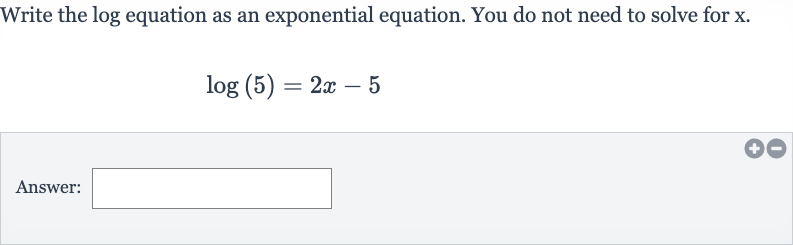
Write the log equation as an exponential equation. You do not need to solve for
x.

log(5)=2x-5
Answer:

Write the log equation as an exponential equation. You do not need to solve for $\mathrm{x}$ . $\newline$ $\log (5)=2 x-5$ $\newline$ Answer:

View step-by-step help

View step-by-step help

Convert between exponential and logarithmic form

Full solution

Q. Write the log equation as an exponential equation. You do not need to solve for

\mathrm{x}

.

\newline

\log (5)=2 x-5

\newline

Answer:

Question Prompt: Question prompt: Convert the logarithmic equation to an exponential equation.
Use Logarithmic Definition: We have the logarithmic equation: $\log(5) = 2x - 5$ . To convert this to an exponential equation, we use the definition of a logarithm. The definition states that if $\log_b(a) = c$ , then $b^c = a$ .
Rewrite as Exponential Equation: Using the definition, we can rewrite the given logarithmic equation as an exponential equation. The base of the logarithm becomes the base of the exponent, the right side of the equation becomes the exponent, and the number inside the log becomes the result of the exponentiation. So, we have $10^{2x - 5} = 5$ , since the base of a common logarithm ( $\log$ ) is $10$ .

More problems from Convert between exponential and logarithmic form

Question

Solve. Write your answer as an integer or a fraction in simplest form.

\newline

5^x=1

\newline

x=

Posted 2 months ago

Question

Steven opened a savings account and deposited

\$100.00

as principal. The account earns

9\%

interest, compounded annually. What is the balance after

5

\newline

Use the formula

A = P(1 + \frac{r}{n})^{nt}

A

is the balance (final amount),

P

is the principal (starting amount),

r

is the interest rate expressed as a decimal,

n

is the number of times per year that the interest is compounded, and

t

is the time in years.

\newline

Round your answer to the nearest cent.

\newline

\$

Posted 2 months ago

Question

Use the following function rule to find

f(1)

\newline

f(x) = 12(7)^x + 8

\newline

f(1) =

Posted 1 month ago

Question

The town of Valley View conducted a census this year, which showed that it has a population of

1,800

people. Based on the census data, it is estimated that the population of Valley View will grow by

12\%

\newline

Write an exponential equation in the form

y = a(b)^x

that can model the town population,

y

x

decades after this census was taken.

\newline

Use whole numbers, decimals, or simplified fractions for the values of

a

b

\newline

y =

Posted 2 months ago

Question

Solve. Round your answer to the nearest thousandth.

\newline

7 = 9^x

\newline

x =

Posted 2 months ago

Question

Solve. Round your answer to the nearest thousandth.

\newline

7 = e^x

\newline

x =

Posted 1 month ago

Question

Solve. Simplify your answer.

\newline

\log u = 1

\newline

u =

Posted 2 months ago

Question

Solve. Simplify your answer.

\newline

7\log x = 7

\newline

x =

Posted 1 month ago

Question

g(t) = 3^t

change over the interval from

t = 3

t = 4

\newline

\newline

g(t)

3

\newline

g(t)

3

\newline

g(t)

3\%

\newline

g(t)

200\%

Posted 2 months ago

Question

f(x)

6

over every unit interval in

x

f(0) = 0

\newline

Which could be a function rule for

f(x)

\newline

\newline

f(x) = 6^x

\newline

f(x) = 6x

\newline

f(x) = \frac{1}{6^x}

\newline

f(x) = \frac{x}{6}

Posted 2 months ago