Resources

Resources

Bytelearn - cat image with glasses

AI tutor

Welcome to Bytelearn!

Let’s check out your problem:

Write the expression below as a single logarithm in simplest form.

2log_(b)9
Answer:
log_(b)(◻)

Write the expression below as a single logarithm in simplest form. $\newline$ $2 \log _{b} 9$ $\newline$ Answer: $\log _{b}(\square)$

View step-by-step help

View step-by-step help

Quotient property of logarithms

Full solution

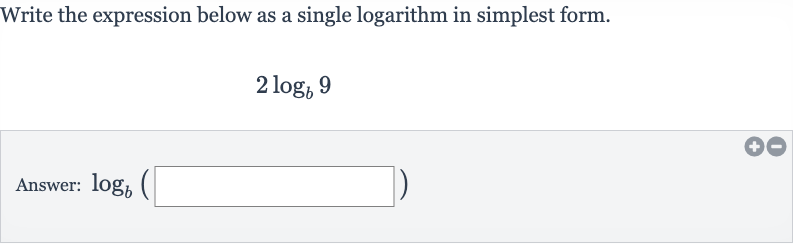
Q. Write the expression below as a single logarithm in simplest form.

\newline

2 \log _{b} 9

\newline

Answer:

\log _{b}(\square)

Question Prompt: Question Prompt: Write the expression $2\log_{b}9$ as a single logarithm in simplest form.
Identify Property: Identify the property used to write the expression as a single logarithm. $\newline$ The expression $2\log_{b}9$ involves a logarithm with a coefficient. To write this as a single logarithm, we use the power property of logarithms. $\newline$ Power Property: $a\log_{b}(P) = \log_{b}(P^{a})$
Apply Power Property: Apply the power property to the given expression. $\newline$ Using the power property, we can move the coefficient $2$ inside the logarithm as an exponent of the argument. $\newline$ $2\log_{b}9 = \log_{b}(9^2)$
Calculate Exponent: Calculate the exponent. $\newline$ Now we calculate $9^2$ to simplify the expression inside the logarithm. $\newline$ $9^2 = 81$
Write Final Expression: Write the final expression. $\newline$ The expression as a single logarithm in simplest form is: $\newline$ $\log_{b}(81)$

More problems from Quotient property of logarithms

Question

Convert the exponential equation in logarithmic form.

\newline

9^3 = 729

Posted 4 months ago

Question

Convert the exponential equation in logarithmic form.

\newline

e^4 \approx 54.598

Posted 4 months ago

Question

Write the logarithmic equation in exponential form.

\newline

\log_{10}100 = 2

\newline

10^2 = \underline{\hspace{2em}}

Posted 9 months ago

Question

Evaluate. Write your answer as a whole number, proper fraction, or improper fraction in simplest form.

\newline

\frac{\ln (e)}{10} =

Posted 4 months ago

Question

Rewrite as a quotient of two common logarithms. Write your answer in simplest form.

\newline

\log_3 33 =

Posted 9 months ago

Question

Evaluate. Round your answer to the nearest thousandth.

\newline

\log_{5}50 =

Posted 9 months ago

Question

Which property of logarithms does this equation demonstrate?

\newline

\log_3 3 + \log_3 6 = \log_3 18

\newline

\newline

\text{Product Property}

\newline

\text{Power Property}

\newline

\text{Quotient Property}

Posted 5 months ago

Question

Expand the logarithm. Assume all expressions exist and are well-defined.

\newline

Write your answer as a sum or difference of common logarithms or multiples of common logarithms. The inside of each logarithm must be a distinct constant or variable.

\newline

\log(uv)

\newline

Posted 9 months ago

Question

Expand the logarithm. Assume all expressions exist and are well-defined.

\newline

Write your answer as a sum or difference of common logarithms or multiples of common logarithms. The inside of each logarithm must be a distinct constant or variable.

\newline

\log v^7

\newline

Posted 9 months ago

Question

Expand the logarithm. Assume all expressions exist and are well-defined.

\newline

Write your answer as a sum or difference of base-

6

logarithms or multiples of base-

6

logarithms. The inside of each logarithm must be a distinct constant or variable.

\newline

\log_6 w^6

\newline

Posted 9 months ago

Related topics

Algebra - Order of Operations Algebra - Distributive Property `X` and `Y` Axes Geometry - Scalene Triangle Common Multiple Geometry - Quadrant