Resources

Resources

Bytelearn - cat image with glasses

AI tutor

Welcome to Bytelearn!

Let’s check out your problem:

Write the expression below as a single logarithm in simplest form.

2log_(b)3-log_(b)3
Answer:
log_(b)(◻)

Write the expression below as a single logarithm in simplest form. $\newline$ $2 \log _{b} 3-\log _{b} 3$ $\newline$ Answer: $\log _{b}(\square)$

View step-by-step help

View step-by-step help

Quotient property of logarithms

Full solution

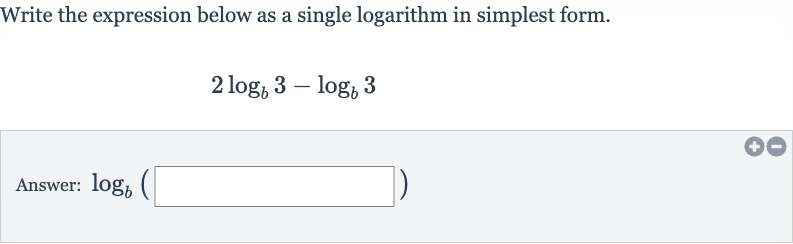
Q. Write the expression below as a single logarithm in simplest form.

\newline

2 \log _{b} 3-\log _{b} 3

\newline

Answer:

\log _{b}(\square)

Understand and Identify: Understand the given expression and identify the logarithm properties to use. $\newline$ We have the expression $2\log_{b}3 - \log_{b}3$ . To combine these logarithms into a single logarithm, we can use the properties of logarithms, specifically the power rule and the subtraction rule. $\newline$ The power rule states that $n\log_{b}(a) = \log_{b}(a^n)$ . $\newline$ The subtraction rule states that $\log_{b}(a) - \log_{b}(c) = \log_{b}(a/c)$ .
Apply Power Rule: Apply the power rule to the first term of the expression. $\newline$ Using the power rule, we can rewrite $2\log_{b}3$ as $\log_{b}(3^2)$ . $\newline$ So, $2\log_{b}3$ becomes $\log_{b}(9)$ .
Combine Using Subtraction Rule: Combine the two logarithms using the subtraction rule. $\newline$ Now we have $\log_{b}(9) - \log_{b}(3)$ . Using the subtraction rule, we can combine these into a single logarithm: $\newline$ $\log_{b}(9) - \log_{b}(3) = \log_{b}\left(\frac{9}{3}\right)$ .
Simplify Fraction: Simplify the fraction inside the logarithm. $\newline$ Simplify $\frac{9}{3}$ to get $3$ . $\newline$ So, $\log_{b}\left(\frac{9}{3}\right)$ becomes $\log_{b}(3)$ .

More problems from Quotient property of logarithms

Question

Solve. Write your answer as an integer or a fraction in simplest form.

\newline

5^x=1

\newline

x=

Posted 2 months ago

Question

Steven opened a savings account and deposited

\$100.00

as principal. The account earns

9\%

interest, compounded annually. What is the balance after

5

\newline

Use the formula

A = P(1 + \frac{r}{n})^{nt}

A

is the balance (final amount),

P

is the principal (starting amount),

r

is the interest rate expressed as a decimal,

n

is the number of times per year that the interest is compounded, and

t

is the time in years.

\newline

Round your answer to the nearest cent.

\newline

\$

Posted 2 months ago

Question

Use the following function rule to find

f(1)

\newline

f(x) = 12(7)^x + 8

\newline

f(1) =

Posted 1 month ago

Question

The town of Valley View conducted a census this year, which showed that it has a population of

1,800

people. Based on the census data, it is estimated that the population of Valley View will grow by

12\%

\newline

Write an exponential equation in the form

y = a(b)^x

that can model the town population,

y

x

decades after this census was taken.

\newline

Use whole numbers, decimals, or simplified fractions for the values of

a

b

\newline

y =

Posted 2 months ago

Question

Solve. Round your answer to the nearest thousandth.

\newline

7 = 9^x

\newline

x =

Posted 2 months ago

Question

Solve. Round your answer to the nearest thousandth.

\newline

7 = e^x

\newline

x =

Posted 1 month ago

Question

Solve. Simplify your answer.

\newline

\log u = 1

\newline

u =

Posted 2 months ago

Question

Solve. Simplify your answer.

\newline

7\log x = 7

\newline

x =

Posted 1 month ago

Question

g(t) = 3^t

change over the interval from

t = 3

t = 4

\newline

\newline

g(t)

3

\newline

g(t)

3

\newline

g(t)

3\%

\newline

g(t)

200\%

Posted 2 months ago

Question

f(x)

6

over every unit interval in

x

f(0) = 0

\newline

Which could be a function rule for

f(x)

\newline

\newline

f(x) = 6^x

\newline

f(x) = 6x

\newline

f(x) = \frac{1}{6^x}

\newline

f(x) = \frac{x}{6}

Posted 2 months ago