Resourcesdown chevron
Testimonials
Plans
Sign in
Sign up
Resourcesright arrow
Testimonials
Plans
Bytelearn - cat image with glassesAI tutor

Welcome to Bytelearn!

Let’s check out your problem:

Write the expression below as a single logarithm in simplest form. 5log_(b)2-log_(b)4 Answer: log_(b)(◻)

Write the expression below as a single logarithm in simplest form.\newline5log⁡b2−log⁡b4 5 \log _{b} 2-\log _{b} 4 \newlineAnswer: log⁡b(□) \log _{b}(\square)

View step-by-step helpView step-by-step help
Homeright chevron icon
Math Problemsright chevron icon
Algebra 2right chevron icon
Quotient property of logarithmsright chevron icon

Full solution

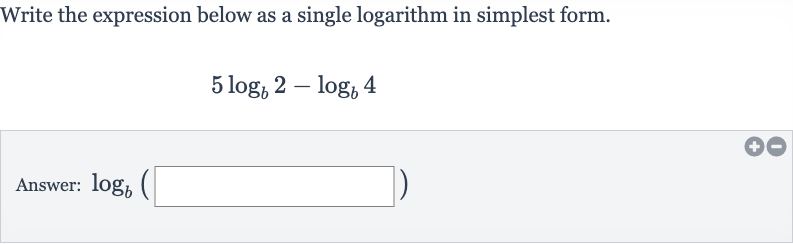
Q. Write the expression below as a single logarithm in simplest form.\newline5log⁡b2−log⁡b4 5 \log _{b} 2-\log _{b} 4 \newlineAnswer: log⁡b(□) \log _{b}(\square)
  1. Recognize Properties: Recognize the properties of logarithms that can be applied.\newlineThe expression given is a combination of two logarithms with the same base that are being subtracted. We can use the power property of logarithms to rewrite the first term and the quotient property to combine the terms into a single logarithm.
  2. Apply Power Property: Apply the power property to the first term.\newlineThe power property of logarithms states that log⁡b(an)=n⋅log⁡b(a)\log_b(a^n) = n \cdot \log_b(a). We can apply this property to the first term to rewrite 5log⁡b25\log_{b}2 as log⁡b(25)\log_{b}(2^5).\newlineCalculation: 25=322^5 = 32
  3. Combine Using Quotient Property: Combine the two logarithms using the quotient property.\newlineThe quotient property of logarithms states that log⁡b(a)−log⁡b(c)=log⁡b(ac)\log_b(a) - \log_b(c) = \log_b\left(\frac{a}{c}\right). We can apply this property to combine log⁡b(32)\log_{b}(32) and log⁡b(4)\log_{b}(4) into a single logarithm.\newlineCalculation: log⁡b(324)\log_{b}\left(\frac{32}{4}\right)
  4. Simplify Inside Logarithm: Simplify the expression inside the logarithm.\newlineWe need to divide 3232 by 44 to simplify the expression inside the logarithm.\newlineCalculation: 32/4=832 / 4 = 8
  5. Write Final Answer: Write the final answer.\newlineThe expression 5log⁡b2−log⁡b45\log_{b}2 - \log_{b}4 can be written as a single logarithm as log⁡b(8)\log_{b}(8).

More problems from Quotient property of logarithms

Question
Write the exponential equation in logarithmic form.\newline93=7299^3 = 729\newlinelog⁡□729=3\log_\square 729 = 3
Get tutor helpright-arrow

Posted 2 months ago

Question
Write the exponential equation in logarithmic form.\newlinee4≈54.598e^4 \approx 54.598\newlineln⁡____≈4\ln\_\_\_\_ \approx 4
Get tutor helpright-arrow

Posted 2 months ago

Question
Write the logarithmic equation in exponential form.\newlinelog⁡10100=2\log_{10}100 = 2\newline102=‾10^2 = \underline{\hspace{2em}}
Get tutor helpright-arrow

Posted 2 months ago

Question
Evaluate. Write your answer as a whole number, proper fraction, or improper fraction in simplest form.\newlineln⁡(e)10=\frac{\ln (e)}{10} = ______
Get tutor helpright-arrow

Posted 2 months ago

Question
Rewrite as a quotient of two common logarithms. Write your answer in simplest form.\newlinelog⁡333=\log_3 33 = ______
Get tutor helpright-arrow

Posted 2 months ago

Question
Evaluate. Round your answer to the nearest thousandth.\newlinelog⁡550=\log_{5}50 = ____
Get tutor helpright-arrow

Posted 2 months ago

Question
Which property of logarithms does this equation demonstrate? \newlinelog⁡33+log⁡36=log⁡318\log_3 3 + \log_3 6 = \log_3 18\newlineChoices:\newline(A) Product Property\text{Product Property}\newline(B) Power Property\text{Power Property}\newline(C) Quotient Property\text{Quotient Property}
Get tutor helpright-arrow

Posted 2 months ago

Question
Expand the logarithm. Assume all expressions exist and are well-defined.\newlineWrite your answer as a sum or difference of common logarithms or multiples of common logarithms. The inside of each logarithm must be a distinct constant or variable.\newlinelog⁡(uv)\log(uv)\newline_____
Get tutor helpright-arrow

Posted 2 months ago

Question
Expand the logarithm. Assume all expressions exist and are well-defined.\newlineWrite your answer as a sum or difference of common logarithms or multiples of common logarithms. The inside of each logarithm must be a distinct constant or variable.\newlinelog⁡v7\log v^7 \newline______
Get tutor helpright-arrow

Posted 2 months ago

Question
Expand the logarithm. Assume all expressions exist and are well-defined.\newlineWrite your answer as a sum or difference of base-66 logarithms or multiples of base-66 logarithms. The inside of each logarithm must be a distinct constant or variable.\newlinelog⁡6w6\log_6 w^6\newline______
Get tutor helpright-arrow

Posted 2 months ago

View all (60)