AI tutor
Full solution
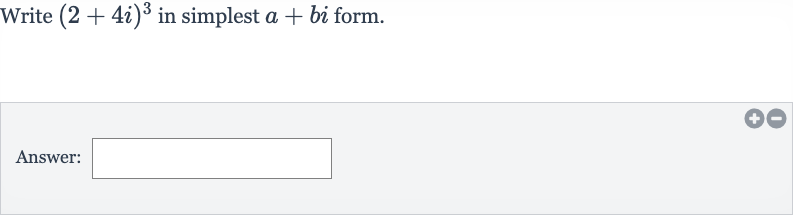
Q. Write in simplest form.Answer:
- Expand Expression: Expand the expression using the binomial theorem or by multiplying by itself three times.Calculation:
- Multiply First Two Terms: Multiply the first two terms together.Calculation: Since , we can simplify this to:
- Multiply Result: Multiply the result from Step by the remaining term.Calculation: (-12 + 16i) \times (2+4i) = -24 + 32i - 48i + 64i^2\(\newlineAgain, since \$i^2 = -1\), we can simplify this to: \(-24 + 32i - 48i - 64 = -88 - 16i\)
- Combine Like Terms: Combine like terms to get the expression in \(a+bi\) form.\(\newline\)Calculation: \(-88 - 16i\)