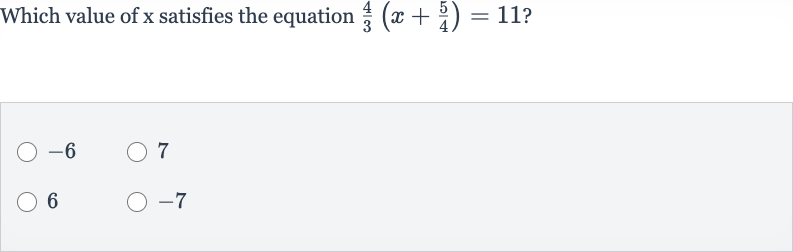Resources

Resources

Bytelearn - cat image with glasses

AI tutor

Welcome to Bytelearn!

Let’s check out your problem:

Which value of
x satisfies the equation
(4)/(3)(x+(5)/(4))=11 ?

-6
7
6

-7

Which value of $x$ satisfies the equation $\frac{4}{3}\left(x+\frac{5}{4}\right)=11$ ? $\newline$ $-6$ $\newline$ $7$ $\newline$ $6$ $\newline$ $-7$

View step-by-step help

View step-by-step help

Solve quadratic inequalities

Full solution

Q. Which value of

x

satisfies the equation

\frac{4}{3}\left(x+\frac{5}{4}\right)=11

?

\newline

-6

\newline

7

\newline

6

\newline

-7

Isolate x Term: Isolate the term containing x. $\newline$ To isolate the term with $x$ , we need to get rid of the fraction by multiplying both sides of the equation by the denominator on the left side, which is $3$ . $\newline$ $(\frac{4}{3})(x + \frac{5}{4}) = 11$ $\newline$ Multiply both sides by $3$ to get: $\newline$ $4(x + \frac{5}{4}) = 11 \times 3$
Multiply Right Side: Perform the multiplication on the right side of the equation. $\newline$ $11 \times 3 = 33$ $\newline$ So now we have: $\newline$ $4(x + \frac{5}{4}) = 33$
Distribute $4$ : Distribute the $4$ on the left side of the equation. $\newline$ $4 \times x = 4x$ $\newline$ $4 \times \left(\frac{5}{4}\right) = 5$ $\newline$ Now the equation looks like this: $\newline$ $4x + 5 = 33$
Subtract $5$ : Subtract $5$ from both sides to isolate the term with $x$ . $\newline$ $4x + 5 - 5 = 33 - 5$ $\newline$ This simplifies to: $\newline$ $4x = 28$
Divide by $4$ : Divide both sides by $4$ to solve for x. $\newline$ $\frac{4x}{4} = \frac{28}{4}$ $\newline$ $x = 7$

More problems from Solve quadratic inequalities

Question

s

\newline

|s| + 3 \geq 8

Posted 4 months ago

Question

Which compound inequality represents the value of

x ?

\newline

(x - 1)(x + 1) > 0

\newline

Posted 4 months ago

Question

A particular company charges advertisers a one time cost of

\$500

, in addition to

\$4.50

for every one thousand times an advertisement is shown on the company's webpage. An advertiser wants its ad to appear

M

thousand times on the webpage, but does not want to spend more than

\$5,000

. Which of the following inequalities best describes the situation?

\newline

\newline

500+4.50M\geq5,000

\newline

4.50+500M>5,000

\newline

500+4.50M\leq5,000

\newline

500+4.50M<5,000

Posted 7 months ago

Question

7x+1 < x+9

\newline

Which of the following best describes the solutions to the inequality shown?

\newline

1

\newline

x > \frac{4}{3}

\newline

x < \frac{4}{3}

\newline

x < 1

\newline

x < \frac{5}{3}

Posted 4 months ago

Question

52-3x < -14

\newline

Which of the following best describes the solutions to the inequality shown?

\newline

1

\newline

x > -\frac{38}{3}

\newline

x > \frac{38}{3}

\newline

x < 22

\newline

x > 22

Posted 10 months ago

Question

5-3x > 2x+2

\newline

Which of the following best describes the solutions to the inequality shown?

\newline

1

\newline

x < \frac{7}{5}

\newline

x < 3

\newline

x < \frac{3}{5}

\newline

x > \frac{3}{5}

Posted 10 months ago

Question

Rani is a real estate agent. For each house she sells, she pays

\$ 100

in fees, but earns a commission of

1.8 \%

of the selling price of the house. Rani's total profit from a particular house is

\$ 4,580

p

represents the selling price of the house, which equation best models the situation?

\newline

1

\newline

0.018 p-100=4580

\newline

0.018 p+100=4580

\newline

(100-0.018) p=4580

\newline

(100+0.018) p=4580

Posted 8 months ago

Question

A commercial airplane that is

1

500

2

500

-mile journey is traveling at

450

knots in still air when it picks up a tailwind of

150

knots (in the same direction). If

h

is the number of hours remaining in the airplane's flight, which of the following equations best describes the situation?

\newline

1

\mathrm{knot}=1.15

miles per hour (mph)

\newline

1

\newline

1,500+690 h=2,500

\newline

1,500+600 h=2,500

\newline

1,500-600 h=2,500

\newline

1,500-690 h=2,500

Posted 7 months ago

Question

A weeping willow that is

15

feet in height grows to a maximum height of

35

y

years at a constant rate of

24

inches per year. Which of the following equations best describes this situation?

\newline

1

=12

\newline

1

\newline

35=15+2 y

\newline

35=15+24 y

\newline

35=15-2 y

\newline

35=15-24 y

Posted 10 months ago

Question

The gas mileage for a car is

23

miles per gallon when the car travels at

60

miles per hour. The car begins a trip with

13

gallons in its tank, travels at an average speed of

60

miles per hour for

h

hours, and ends the trip with

10

gallons in its tank. Which of the following equations best models this situation?

\newline

1

\newline

13-\frac{23 h}{60}=10

\newline

13-\frac{60 h}{23}=10

\newline

\frac{13-60 h}{23}=10

\newline

\frac{13-23 h}{60}=10

Posted 8 months ago

Related topics

Algebra - Order of Operations Algebra - Distributive Property `X` and `Y` Axes Geometry - Scalene Triangle Common Multiple Geometry - Quadrant