Full solution
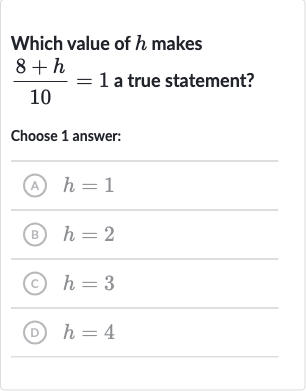
Q. Which value of makes a true statement?Choose answer:(A) (B) (C) (D)
- Multiply both sides by : To find the value of that makes the equation true, we need to isolate on one side of the equation. We start by multiplying both sides of the equation by to eliminate the denominator.
- Simplify the equation: After multiplying both sides by , we get:
- Subtract from both sides: Now, we subtract from both sides to solve for .
- Solve for h: This simplifies to:
- Check the solution: We check our solution by substituting back into the original equation to see if it makes the equation true.