Resourcesdown chevron
Testimonials
Plans
Sign in
Sign up
Resourcesright arrow
Testimonials
Plans
Bytelearn - cat image with glassesAI tutor

Welcome to Bytelearn!

Let’s check out your problem:

Which of the following is equivalent to log((a)/(b)). a)(log a)/(log b) b)log(a-b) c)log a-log b d)log b-log a

Which of the following is equivalent to log⁡(ab)\log\left(\frac{a}{b}\right).\newlinea) log⁡alog⁡b\frac{\log a}{\log b}\newlineb) log⁡(a−b)\log(a-b)\newlinec) log⁡a−log⁡b\log a-\log b\newlined) log⁡b−log⁡a\log b-\log a

View step-by-step helpView step-by-step help
Homeright chevron icon
Math Problemsright chevron icon
Algebra 2right chevron icon
Quotient property of logarithmsright chevron icon

Full solution

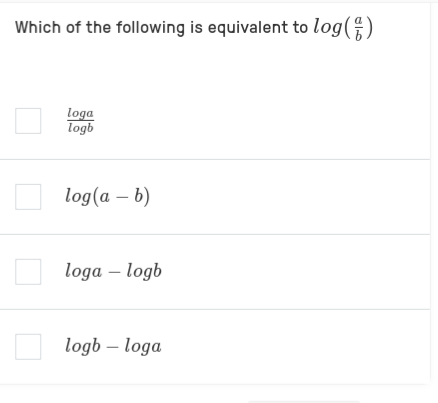
Q. Which of the following is equivalent to log⁡(ab)\log\left(\frac{a}{b}\right).\newlinea) log⁡alog⁡b\frac{\log a}{\log b}\newlineb) log⁡(a−b)\log(a-b)\newlinec) log⁡a−log⁡b\log a-\log b\newlined) log⁡b−log⁡a\log b-\log a
  1. Identify Property: Identify the property used to expand log⁡(ab)\log\left(\frac{a}{b}\right). Using the quotient property of logarithms, which states log⁡b(PQ)=log⁡bP−log⁡bQ\log_b\left(\frac{P}{Q}\right) = \log_b P - \log_b Q.
  2. Apply Quotient Property: Apply the quotient property to log⁡(ab)\log\left(\frac{a}{b}\right).\newlinelog⁡(ab)=log⁡a−log⁡b\log\left(\frac{a}{b}\right) = \log a - \log b.
  3. Compare with Options: Compare the expanded form with the given options.\newlineThe correct form log⁡a−log⁡b\log a - \log b matches with one of the options.

More problems from Quotient property of logarithms

Question
Convert the exponential equation in logarithmic form.\newline93=7299^3 = 729
Get tutor helpright-arrow

Posted 4 months ago

Question
Convert the exponential equation in logarithmic form.\newlinee4≈54.598e^4 \approx 54.598
Get tutor helpright-arrow

Posted 4 months ago

Question
Write the logarithmic equation in exponential form.\newlinelog⁡10100=2\log_{10}100 = 2\newline102=‾10^2 = \underline{\hspace{2em}}
Get tutor helpright-arrow

Posted 9 months ago

Question
Evaluate. Write your answer as a whole number, proper fraction, or improper fraction in simplest form.\newlineln⁡(e)10=\frac{\ln (e)}{10} = ______
Get tutor helpright-arrow

Posted 4 months ago

Question
Rewrite as a quotient of two common logarithms. Write your answer in simplest form.\newlinelog⁡333=\log_3 33 = ______
Get tutor helpright-arrow

Posted 9 months ago

Question
Evaluate. Round your answer to the nearest thousandth.\newlinelog⁡550=\log_{5}50 = ____
Get tutor helpright-arrow

Posted 9 months ago

Question
Which property of logarithms does this equation demonstrate? \newlinelog⁡33+log⁡36=log⁡318\log_3 3 + \log_3 6 = \log_3 18\newlineChoices:\newline(A) Product Property\text{Product Property}\newline(B) Power Property\text{Power Property}\newline(C) Quotient Property\text{Quotient Property}
Get tutor helpright-arrow

Posted 5 months ago

Question
Expand the logarithm. Assume all expressions exist and are well-defined.\newlineWrite your answer as a sum or difference of common logarithms or multiples of common logarithms. The inside of each logarithm must be a distinct constant or variable.\newlinelog⁡(uv)\log(uv)\newline_____
Get tutor helpright-arrow

Posted 9 months ago

Question
Expand the logarithm. Assume all expressions exist and are well-defined.\newlineWrite your answer as a sum or difference of common logarithms or multiples of common logarithms. The inside of each logarithm must be a distinct constant or variable.\newlinelog⁡v7\log v^7 \newline______
Get tutor helpright-arrow

Posted 9 months ago

Question
Expand the logarithm. Assume all expressions exist and are well-defined.\newlineWrite your answer as a sum or difference of base-66 logarithms or multiples of base-66 logarithms. The inside of each logarithm must be a distinct constant or variable.\newlinelog⁡6w6\log_6 w^6\newline______
Get tutor helpright-arrow

Posted 9 months ago

View all (54)

Related topics

Algebra - Order of OperationsAlgebra - Distributive Property`X` and `Y` AxesGeometry - Scalene TriangleCommon MultipleGeometry - Quadrant