Full solution
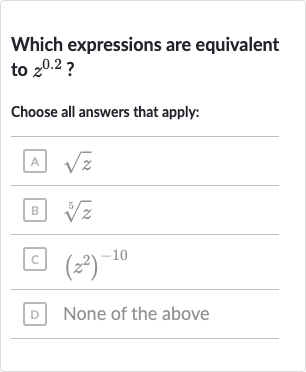
Q. Which expressions are equivalent to ?Choose all answers that apply:A B C D None of the above
- Understand Expression: Understand the given expression . The given expression can be rewritten as the th root of because is the same as . So, .
- Compare Option A: Compare the given expression to option A.Option A is , which is the same as . This is not equivalent to because is not equal to .
- Compare Option B: Compare the given expression to option B.Option B is , which is the same as . This is equivalent to because is equal to .
- Compare Option C: Compare the given expression to option C.Option C is . Using the power of a power rule, we can simplify this expression as . This is not equivalent to because is not equal to .
- Determine Option D: Determine if option D is correct.Option D states "None of the above," which is not correct because we have already established that option B is equivalent to .