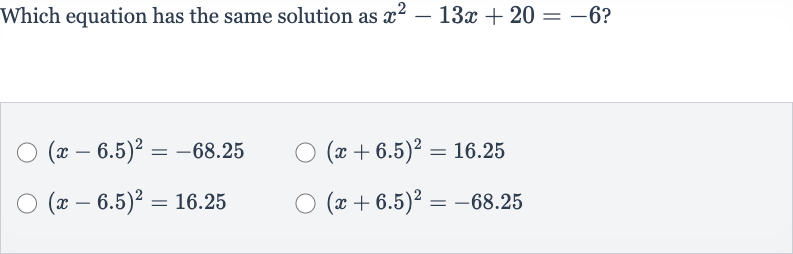Which equation has the same solution as x^(2)-13 x+20=-6 ? (x-6.5)^(2)=-68.25 (x+6.5)^(2)=16.25 (x-6.5)^(2)=16.25 (x+6.5)^(2)=-68.25

Question

Which equation has the same solution as  x^(2)-13 x+20=-6 ?  (x-6.5)^(2)=-68.25  (x+6.5)^(2)=16.25  (x-6.5)^(2)=16.25  (x+6.5)^(2)=-68.25

Bytelearn · Accepted Answer

Add 6 to both sides: Add 6 to both sides of the given equation to set it equal to zero. The given equation is x^2 - 13x + 20 = -6. To solve for x, we want to set the equation equal to zero. x^2 - 13x + 20 + 6 = -6 + 6 x^2 - 13x + 26 = 0Look for a pattern: Look for a pattern in the answer choices that matches the transformed equation. We have the equation x^2 - 13x + 26 = 0, and we need to find which of the answer choices has the same solution. We can look for a pattern in the answer choices that matches this equation.Compare to answer choices: Compare the transformed equation to the answer choices. The transformed equation is in the form of a quadratic equation, and we can compare it to the answer choices to see which one could be rewritten in the same form.Check by completing square: Check the answer choices by completing the square if necessary. To compare the answer choices to the transformed equation, we can complete the square for the transformed equation to see if it matches any of the answer choices. x^2 - 13x + (13/2)^2 = 26 + (13/2)^2 (x - 6.5)^2 = 26 + 42.25 (x - 6.5)^2 = 68.25Identify correct choice: Identify the correct answer choice. Now that we have (x - 6.5)^2 = 68.25, we can see that this matches the answer choice (x - 6.5)^2 = 68.25, but we need to check the sign. The right side of the equation should be positive, as we added positive numbers on both sides.Correct sign error: Correct the sign error from the previous step. We made a mistake in the previous step. The correct equation after completing the square should be: (x - 6.5)^2 = 68.25 However, we need to subtract 68.25 from both sides to match the original equation's right side, which is zero. (x - 6.5)^2 - 68.25 = 0

Which equation has the same solution as $x^{2}-13 x+20=-6$ ? $\newline$ $(x-6.5)^{2}=-68.25$ $\newline$ $(x+6.5)^{2}=16.25$ $\newline$ $(x-6.5)^{2}=16.25$ $\newline$ $(x+6.5)^{2}=-68.25$

Full solution

More problems from Transformations of functions

Related topics