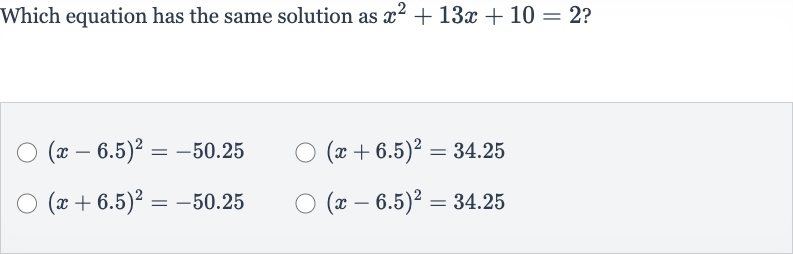AI tutor
Full solution
Q. Which equation has the same solution as ?
- Simplify Equation: Simplify the given equation by moving all terms to one side to set the equation equal to zero.
- Factor Quadratic: Look for a way to factor the quadratic equation, if possible.We need to find two numbers that multiply to and add up to . However, there are no such integer pairs, so we might need to complete the square or use the quadratic formula to solve for .
- Complete the Square: Complete the square for the quadratic equation .First, move the constant term to the other side:Next, take half of the coefficient of , which is , square it, and add it to both sides of the equation:
- Convert to Decimal: Convert the fraction to a decimal to compare with the given choices.So, the equation becomes:
- Compare with Choices: Compare the completed square form of the equation with the given choices.We have , and since is , the equation can be written as:This matches one of the given choices.
More problems from Transformations of functions
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help