Resourcesdown chevron
Testimonials
Plans
Sign in
Sign up
Resourcesright arrow
Testimonials
Plans
Bytelearn - cat image with glassesAI tutor

Welcome to Bytelearn!

Let’s check out your problem:

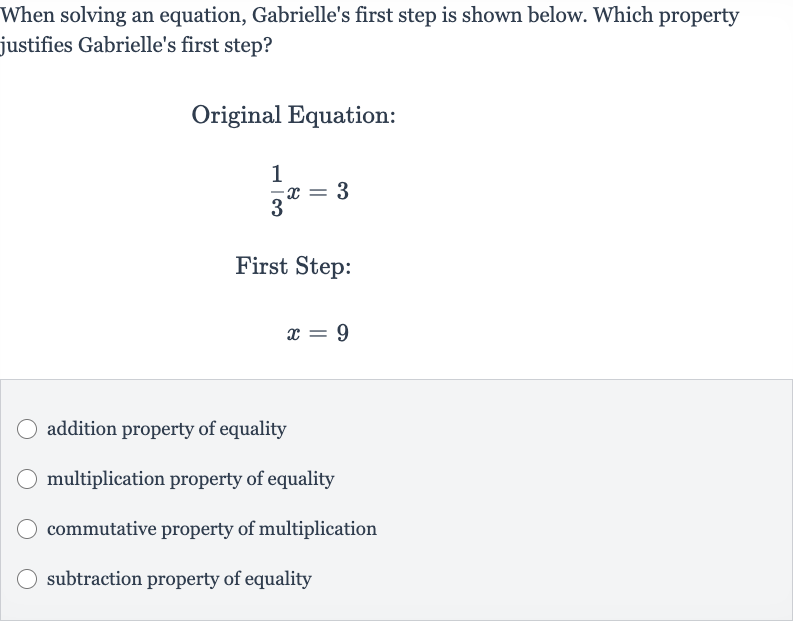
When solving an equation, Gabrielle's first step is shown below. Which property justifies Gabrielle's first step? Original Equation: (1)/(3)x=3 First Step: x=9 addition property of equality multiplication property of equality commutative property of multiplication subtraction property of equality

When solving an equation, Gabrielle's first step is shown below. Which property justifies Gabrielle's first step?\newlineOriginal Equation:\newline13x=3 \frac{1}{3} x=3 \newlineFirst Step:\newlinex=9 x=9 \newlineaddition property of equality\newlinemultiplication property of equality\newlinecommutative property of multiplication\newlinesubtraction property of equality

View step-by-step helpView step-by-step help
Homeright chevron icon
Math Problemsright chevron icon
Algebra 2right chevron icon
Identify properties of logarithmsright chevron icon

Full solution

Q. When solving an equation, Gabrielle's first step is shown below. Which property justifies Gabrielle's first step?\newlineOriginal Equation:\newline13x=3 \frac{1}{3} x=3 \newlineFirst Step:\newlinex=9 x=9 \newlineaddition property of equality\newlinemultiplication property of equality\newlinecommutative property of multiplication\newlinesubtraction property of equality
  1. Initial Equation: Gabrielle's first step in solving the equation is to go from (13)x=3(\frac{1}{3})x = 3 to x=9x = 9. To justify this step, we need to determine which property allows us to go from the original equation to the first step. Let's analyze the step:\newlineOriginal Equation: (13)x=3(\frac{1}{3})x = 3\newlineFirst Step: x=9x = 9\newlineTo isolate xx, we need to eliminate the fraction (13)(\frac{1}{3}) that is being multiplied by xx. To do this, we can multiply both sides of the equation by the reciprocal of (13)(\frac{1}{3}), which is 33. This is because multiplying by the reciprocal will cancel out the (13)(\frac{1}{3}) on the left side, leaving us with just xx.\newlineCalculation:\newlinex=9x = 911\newlinex=9x = 9\newlineThis step is justified by the multiplication property of equality, which states that if you multiply both sides of an equation by the same nonzero number, the two sides remain equal.

More problems from Identify properties of logarithms

Question
Write the exponential equation in logarithmic form.\newline93=7299^3 = 729\newlinelog⁡□729=3\log_\square 729 = 3
Get tutor helpright-arrow

Posted 2 months ago

Question
Write the exponential equation in logarithmic form.\newlinee4≈54.598e^4 \approx 54.598\newlineln⁡____≈4\ln\_\_\_\_ \approx 4
Get tutor helpright-arrow

Posted 2 months ago

Question
Write the logarithmic equation in exponential form.\newlinelog⁡10100=2\log_{10}100 = 2\newline102=‾10^2 = \underline{\hspace{2em}}
Get tutor helpright-arrow

Posted 2 months ago

Question
Evaluate. Write your answer as a whole number, proper fraction, or improper fraction in simplest form.\newlineln⁡(e)10=\frac{\ln (e)}{10} = ______
Get tutor helpright-arrow

Posted 2 months ago

Question
Rewrite as a quotient of two common logarithms. Write your answer in simplest form.\newlinelog⁡333=\log_3 33 = ______
Get tutor helpright-arrow

Posted 2 months ago

Question
Evaluate. Round your answer to the nearest thousandth.\newlinelog⁡550=\log_{5}50 = ____
Get tutor helpright-arrow

Posted 2 months ago

Question
Which property of logarithms does this equation demonstrate? \newlinelog⁡33+log⁡36=log⁡318\log_3 3 + \log_3 6 = \log_3 18\newlineChoices:\newline(A) Product Property\text{Product Property}\newline(B) Power Property\text{Power Property}\newline(C) Quotient Property\text{Quotient Property}
Get tutor helpright-arrow

Posted 2 months ago

Question
Expand the logarithm. Assume all expressions exist and are well-defined.\newlineWrite your answer as a sum or difference of common logarithms or multiples of common logarithms. The inside of each logarithm must be a distinct constant or variable.\newlinelog⁡(uv)\log(uv)\newline_____
Get tutor helpright-arrow

Posted 2 months ago

Question
Expand the logarithm. Assume all expressions exist and are well-defined.\newlineWrite your answer as a sum or difference of common logarithms or multiples of common logarithms. The inside of each logarithm must be a distinct constant or variable.\newlinelog⁡v7\log v^7 \newline______
Get tutor helpright-arrow

Posted 2 months ago

Question
Expand the logarithm. Assume all expressions exist and are well-defined.\newlineWrite your answer as a sum or difference of base-66 logarithms or multiples of base-66 logarithms. The inside of each logarithm must be a distinct constant or variable.\newlinelog⁡6w6\log_6 w^6\newline______
Get tutor helpright-arrow

Posted 2 months ago

View all (19)