AI tutor
Full solution
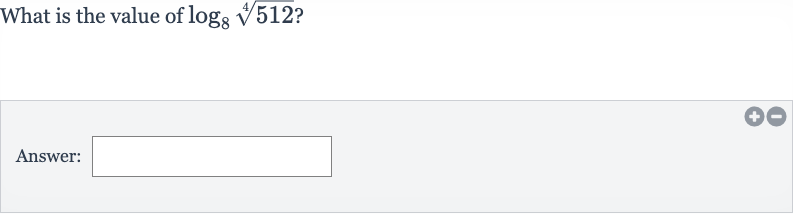
Q. Evaluate.Write your answer in simplest form.
- Identify Fourth Root of : Identify the value of the fourth root of .The fourth root of is the number that, when raised to the power of , equals . We can calculate this as ., so .Since is the fourth root of , and the fourth root of is (approximately), we have (approximately).However, we know that is exactly , so the fourth root of is exactly .
- Apply Logarithm: Apply the logarithm to the fourth root of . We need to find , which we can write as .
- Convert Base to : Convert the base of the logarithm from to . Since is , we can use the change of base formula to convert the base from to . The change of base formula is . So, .
- Calculate Log Values: Calculate and . We know that is , so . We also know that is , so .
- Divide Logarithms: Divide the two logarithms to find the value.Now we divide the results from Step to find the value of .