AI tutor
Full solution
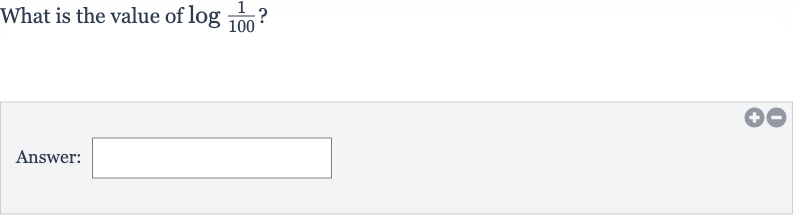
Q. Evaluate.Write your answer in simplest form.
- Identify Base: Question prompt: What is the value of ?
- Apply Definition: Recognize the base of the logarithm.The logarithm given does not specify a base, which means it is a common logarithm and has a base of .
- Express as Power: Apply the definition of a common logarithm.The common logarithm of a number is the power to which the base must be raised to obtain that number.
- Rewrite Logarithm: Express as a power of . is equal to .
- Apply Power Rule: Rewrite the logarithm using the power of . can be rewritten as because is the same as to the power of .
- Evaluate Logarithm: Apply the power rule of logarithms.The power rule of logarithms states that . Therefore, .
- Multiply Exponent: Evaluate . Since the base of the logarithm is , is equal to .
- Multiply Exponent: Evaluate . Since the base of the logarithm is , is equal to .Multiply the exponent by the value of . is equal to , which simplifies to .