Resources

Resources

Bytelearn - cat image with glasses

AI tutor

Welcome to Bytelearn!

Let’s check out your problem:

What is the total number of different 10-letter arrangements that can be formed using the letters in the word COMMISSION?
Answer:

What is the total number of different $10$ -letter arrangements that can be formed using the letters in the word COMMISSION? $\newline$ Answer:

View step-by-step help

View step-by-step help

Write a linear function: word problems

Full solution

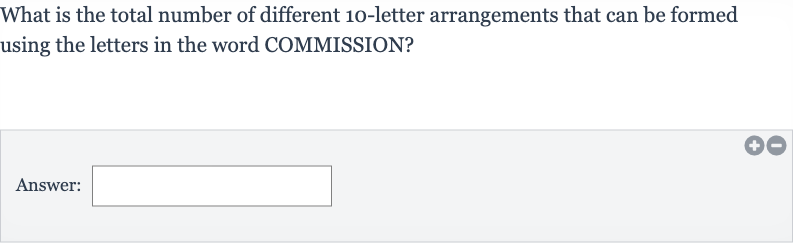
Q. What is the total number of different

10

-letter arrangements that can be formed using the letters in the word COMMISSION?

\newline

Answer:

Count Letters: Count the number of each letter in COMMISSION. $\newline$ $C=1$ , $O=2$ , $M=2$ , $I=2$ , $S=2$ , $N=1$ .
Calculate Total Factorial: Calculate the factorial of the total number of letters. $10! = 10 \times 9 \times 8 \times 7 \times 6 \times 5 \times 4 \times 3 \times 2 \times 1$ .
Divide by Repeating Factorials: Divide by the factorial of the number of times each letter repeats to correct for overcounting. $\newline$ The equation is $10! / (2! \times 2! \times 2! \times 2!)$ .
Do the Math: Do the math: $\frac{10!}{(2! \times 2! \times 2! \times 2!)} = \frac{(10 \times 9 \times 8 \times 7 \times 6 \times 5 \times 4 \times 3 \times 2 \times 1)}{(2 \times 2 \times 2 \times 2)}$ .
Simplify Equation: Simplify the equation: $\frac{10!}{(2! \times 2! \times 2! \times 2!)} = \frac{(10 \times 9 \times 8 \times 7 \times 6 \times 5 \times 4 \times 3)}{(2 \times 2 \times 2)}$ .
Calculate Result: Calculate the result: $(10 \times 9 \times 8 \times 7 \times 6 \times 5 \times 4 \times 3) / (2 \times 2 \times 2) = 453600$ .

More problems from Write a linear function: word problems

Question

This equation shows how the time required to screen-print a batch of shirts is related to the number of shirts in the batch.

\newline

t = s + 13

\newline

s

represents the number of shirts in the batch, and the variable

t

represents the time required to screen-print the shirts. How long does it take to screen-print a batch of

4

\newline

_________minutes

Posted 2 months ago

Question

Krysta wants to take piano lessons. She asked a teacher how many new pieces she could expect to learn each month. The teacher gave an estimate of

3

. Write an equation that shows how the total number of pieces Krysta would learn,

y

, depends on the number of months of piano lessons,

x

\newline

y

Posted 2 months ago

Question

Tom bought a boat for

\$3,000

and incurs a daily storage fee of

\$15

. Write a linear function to represent the total amount Tom will owe,

p(x)

x

days to pick it up.

\newline

Posted 3 months ago

Question

180

-page book cover to cover in a single session, at a constant rate. It took him

6

\newline

\newline

y

represent the number of pages left to read after

\newline

t

\newline

Which of the following information about the graph of the relationship is given?

\newline

1

\newline

\newline

x

\newline

\newline

y

\newline

(C) Slope and a point that is not an intercept

\newline

\newline

x

\newline

y

\newline

\newline

y

-intercept and a point that is not an intercept

\newline

(F) Two points that are not intercepts

Posted 4 months ago

Question

A charity organization had to sell

18

tickets to their fundraiser just to cover necessary production costs. They sold each ticket for

\$45

y

represent the net profit (in dollars) when they have sold

x

tickets. Which of the following information about the graph of the relationship is given?

\newline

1

\newline

x

\newline

y

\newline

(C) Slope and a point that is not an intercept

\newline

x

y

\newline

y

-intercept and a point that is not an intercept

\newline

(F) Two points that are not intercepts

Posted 2 months ago

Question

Hiro painted his room. After

3

hours of painting at a rate of

8

square meters per hour, he had

28

square meters left to paint.

\newline

\newline

y

represent the area (in square meters) left to paint after

\newline

x

\newline

Which of the following information about the graph of the relationship is given?

\newline

1

\newline

\newline

x

\newline

\newline

y

\newline

(C) Slope and a point that is not an intercept

\newline

\newline

x

\newline

y

\newline

\newline

y

-intercept and a point that is not an intercept

\newline

(F) Two points that are not intercepts

Posted 2 months ago

Question

Naoya read a book cover to cover in a single session, at a rate of

55

pages per hour. After

4

350

pages left to read.

\newline

y

represent the number of pages left to read after

x

\newline

Complete the equation for the relationship between the number of pages left and number of hours.

\newline

y=

Posted 1 month ago

Question

A teacher purchased

300

colored pencils for an upcoming project. The pencils she ordered come in packs of

20

30

14

packs in all. Write a system of equations to find the number of

20

(x)

30

(y)

the art teacher ordered.

Posted 2 months ago

Question

C=0.25(L-1200)

\newline

C

, in dollars, to the holder of a liability insurance account given a total liability of

L

dollars is given by the equation as long as

C

is positive. By how many dollars does the liability increase when the cost to the holder increases by

1

Posted 2 months ago

Question

P=\frac{2}{5} m+1.5

\newline

P

, in dollars, of a train ticket used to travel a distance of

m

miles is given by the equation. By how many miles does the traveling distance increase if the ticket price increases by

1

Posted 4 months ago