Resources

Resources

Bytelearn - cat image with glasses

AI tutor

Welcome to Bytelearn!

Let’s check out your problem:

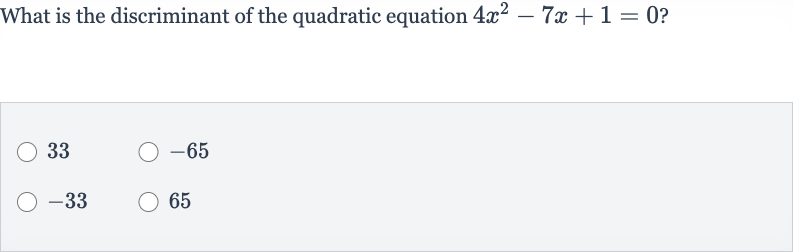
What is the discriminant of the quadratic equation
4x^(2)-7x+1=0 ?
33

-65

-33
65

What is the discriminant of the quadratic equation $4 x^{2}-7 x+1=0$ ? $\newline$ $33$ $\newline$ $-65$ $\newline$ $-33$ $\newline$ $65$

View step-by-step help

View step-by-step help

Solve exponential equations by rewriting the base

Full solution

Q. What is the discriminant of the quadratic equation

4 x^{2}-7 x+1=0

?

\newline

33

\newline

-65

\newline

-33

\newline

65

Identify Coefficients: The discriminant of a quadratic equation $ax^2 + bx + c = 0$ is given by the formula $D = b^2 - 4ac$ . Let's identify the coefficients $a$ , $b$ , and $c$ from the given equation $4x^2 - 7x + 1 = 0$ .
Calculate Coefficients: The coefficient $a$ is the coefficient of $x^2$ , which is $4$ . The coefficient $b$ is the coefficient of $x$ , which is $-7$ . The coefficient $c$ is the constant term, which is $1$ .
Plug into Formula: Now we will plug these values into the discriminant formula $D = b^2 - 4ac$ . So we have $D = (-7)^2 - 4(4)(1)$ .
Calculate Discriminant: Calculating the discriminant, we get $D = 49 - 16$ . We square $-7$ to get $49$ and multiply $4$ by $4$ and then by $1$ to get $16$ .
Final Result: Subtracting $16$ from $49$ , we get $D = 33$ . This is the value of the discriminant.

More problems from Solve exponential equations by rewriting the base

Question

Solve. Write your answer as an integer or a fraction in simplest form.

\newline

5^x=1

\newline

x=

Posted 2 months ago

Question

Steven opened a savings account and deposited

\$100.00

as principal. The account earns

9\%

interest, compounded annually. What is the balance after

5

\newline

Use the formula

A = P(1 + \frac{r}{n})^{nt}

A

is the balance (final amount),

P

is the principal (starting amount),

r

is the interest rate expressed as a decimal,

n

is the number of times per year that the interest is compounded, and

t

is the time in years.

\newline

Round your answer to the nearest cent.

\newline

\$

Posted 2 months ago

Question

Use the following function rule to find

f(1)

\newline

f(x) = 12(7)^x + 8

\newline

f(1) =

Posted 1 month ago

Question

The town of Valley View conducted a census this year, which showed that it has a population of

1,800

people. Based on the census data, it is estimated that the population of Valley View will grow by

12\%

\newline

Write an exponential equation in the form

y = a(b)^x

that can model the town population,

y

x

decades after this census was taken.

\newline

Use whole numbers, decimals, or simplified fractions for the values of

a

b

\newline

y =

Posted 2 months ago

Question

Solve. Round your answer to the nearest thousandth.

\newline

7 = 9^x

\newline

x =

Posted 2 months ago

Question

Solve. Round your answer to the nearest thousandth.

\newline

7 = e^x

\newline

x =

Posted 1 month ago

Question

Solve. Simplify your answer.

\newline

\log u = 1

\newline

u =

Posted 2 months ago

Question

Solve. Simplify your answer.

\newline

7\log x = 7

\newline

x =

Posted 1 month ago

Question

g(t) = 3^t

change over the interval from

t = 3

t = 4

\newline

\newline

g(t)

3

\newline

g(t)

3

\newline

g(t)

3\%

\newline

g(t)

200\%

Posted 2 months ago

Question

f(x)

6

over every unit interval in

x

f(0) = 0

\newline

Which could be a function rule for

f(x)

\newline

\newline

f(x) = 6^x

\newline

f(x) = 6x

\newline

f(x) = \frac{1}{6^x}

\newline

f(x) = \frac{x}{6}

Posted 2 months ago