Resourcesdown chevron
Testimonials
Plans
Sign in
Sign up
Resourcesright arrow
Testimonials
Plans
Bytelearn - cat image with glassesAI tutor

Welcome to Bytelearn!

Let’s check out your problem:

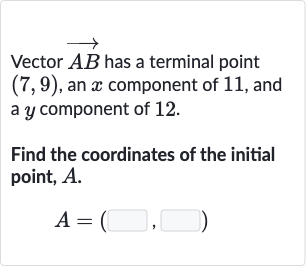
Vector vec(AB) has a terminal point (7,9), an x component of 11 , and a y component of 12 . Find the coordinates of the initial point, A. A=(◻,◻)

Vector ABundefined \overrightarrow{A B} has a terminal point (7,9) (7,9) , an x x component of 1111 , and a y y component of 1212 .\newlineFind the coordinates of the initial point, A A .\newlineA=(□,□) A=(\square, \square)

View step-by-step helpView step-by-step help
Homeright chevron icon
Math Problemsright chevron icon
Algebra 2right chevron icon
Write equations of cosine functions using propertiesright chevron icon

Full solution

Q. Vector ABundefined \overrightarrow{A B} has a terminal point (7,9) (7,9) , an x x component of 1111 , and a y y component of 1212 .\newlineFind the coordinates of the initial point, A A .\newlineA=(□,□) A=(\square, \square)
  1. Find Initial Point A: To find the initial point AA of the vector AB⃗\vec{AB}, we need to subtract the vector's components from the terminal point's coordinates.\newlineGiven:\newlineTerminal point B=(7,9)B = (7, 9)\newlinex component of AB⃗=11\vec{AB} = 11\newliney component of AB⃗=12\vec{AB} = 12\newlineThe initial point AA can be found using the following formulas:\newlineAx=Bx−AB⃗xA_x = B_x - \vec{AB}_x\newlineAy=By−AB⃗yA_y = B_y - \vec{AB}_y
  2. Calculate AxA_x: Calculate the x-coordinate of the initial point A using the formula:\newlineAx=Bx−AB⃗xA_x = B_x - \vec{AB}_x\newlineAx=7−11A_x = 7 - 11\newlineAx=−4A_x = -4
  3. Calculate AyA_y: Calculate the y-coordinate of the initial point AA using the formula:\newlineAy=By−AB⃗yA_y = B_y - \vec{AB}_y\newlineAy=9−12A_y = 9 - 12\newlineAy=−3A_y = -3

More problems from Write equations of cosine functions using properties

Question
Find an equation for a sinusoidal function that has period 2π2\pi, amplitude 11, and contains the point (π,−1)\left(\pi, -1\right). \newlineWrite your answer in the form f(x)=Acos⁡(Bx+C)+Df(x)=A\cos(Bx+C)+D, where AA, BB, CC, and DD are real numbers.\newline f(x)=f(x)=
Get tutor helpright-arrow

Posted 7 months ago

Question
Find an equation for a sinusoidal function that has period 2π2\pi, amplitude 11, and contains the point (−π2,−1)\left(-\frac{\pi}{2},-1\right). Write your answer in the form f(x)=Asin⁡(Bx+C)+Df(x) = A\sin(Bx + C) + D, where AA, BB, CC, and DD are real numbers. `f(x)` =______
Get tutor helpright-arrow

Posted 9 months ago

Question
Find an equation for a sinusoidal function that has period 2π2\pi, amplitude 11, and contains the point (0,−1)(0,-1). \newlineWrite your answer in the form f(x)=Acos⁡(Bx+C)+Df(x) = A\cos(Bx + C) + D, where AA, BB, CC, and DD are real numbers. \newlinef(x)=f(x) = _____
Get tutor helpright-arrow

Posted 8 months ago

Question
Franklin Middle School is having a canned food drive. In Mr. Lao's class, Mr. Lao brought 1010 cans of food, and each student brought 22 cans. In Ms. Roger's class, Ms. Rogers did not bring any cans, but each student brought 33 cans of food. Write two equations in slope-intercept form to represent the number of cans of food for each class yy in terms of the number of students xx.
Get tutor helpright-arrow

Posted 8 months ago

Question
Franklin Middle School is having a canned food drive. In Mr. Lao's class, Mr. Lao brought 1010 cans of food, and each student brought 22 cans. In Ms. Roger's class, Ms. Rogers did not bring any cans, but each student brought 33 cans of food. Write two equations in slope-intercept form to represent the number of cans of food for each class yy in terms of the number of students xx.
Get tutor helpright-arrow

Posted 8 months ago

Question
Kris is wrapping Christmas lights around the railing that runs around three sides of his square porch If one side of his porch is 1212 feet long, and each strand of his Christmes lights will wrap around a 7070-inch length of the railing, what is the minimum number of strands Kris needs to completely wrap the porch railing?\newlineA) 66\newlineB) 77\newlineC) 88\newlineD) 99
Get tutor helpright-arrow

Posted 8 months ago

Question
Kris is wrapping Christmas lights around the railing that runs around three sides of his square porch If one side of his porch is 1212 feet long, and each strand of his Christmas lights will wrap around a 7070-inch length of the railing, what is the minimum number of strands Kris needs to completely wrap the porch railing?\newlineA) 66\newlineB) 77\newlineC) 88\newlineD) 99
Get tutor helpright-arrow

Posted 8 months ago

Question
For a given input value b b , the function f f outputs a value a a to satisfy the following equation.\newline4a+7b=−52 4a+7b=-52 \newlineWrite a formula for f(b) f(b) in terms of b b .\newlinef(b)=□ f(b)=\square
Get tutor helpright-arrow

Posted 6 months ago

Question
For a given input value xx, the function gg outputs a value yy to satisfy the following equation.\newline−4x−6=−5y+2-4x-6=-5y+2\newlineWrite a formula for g(x)g(x) in terms of xx.\newlineg(x)=□g(x)=\square
Get tutor helpright-arrow

Posted 10 months ago

Question
For a given input value aa, the function ff outputs a value bb to satisfy the following equation.\newline−3a+6b=a+4b-3a+6b=a+4b\newlineWrite a formula for f(a)f(a) in terms of aa.\newlinef(a)=□f(a)=\square
Get tutor helpright-arrow

Posted 10 months ago

View all (68)

Related topics

Algebra - Order of OperationsAlgebra - Distributive Property`X` and `Y` AxesGeometry - Scalene TriangleCommon MultipleGeometry - Quadrant