Resourcesdown chevron
Testimonials
Plans
Sign in
Sign up
Resourcesright arrow
Testimonials
Plans
Bytelearn - cat image with glassesAI tutor

Welcome to Bytelearn!

Let’s check out your problem:

For a given input value a, the function f outputs a value b to satisfy the following equation. -3a+6b=a+4b Write a formula for f(a) in terms of a. f(a)=◻

For a given input value aa, the function ff outputs a value bb to satisfy the following equation.\newline−3a+6b=a+4b-3a+6b=a+4b\newlineWrite a formula for f(a)f(a) in terms of aa.\newlinef(a)=□f(a)=\square

View step-by-step helpView step-by-step help
Homeright chevron icon
Math Problemsright chevron icon
Algebra 2right chevron icon
Write equations of cosine functions using propertiesright chevron icon

Full solution

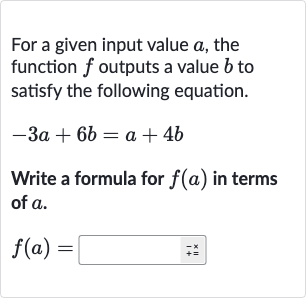
Q. For a given input value aa, the function ff outputs a value bb to satisfy the following equation.\newline−3a+6b=a+4b-3a+6b=a+4b\newlineWrite a formula for f(a)f(a) in terms of aa.\newlinef(a)=□f(a)=\square
  1. Isolate bb on one side: To solve for bb in terms of aa, we need to isolate bb on one side of the equation. Let's start by moving all terms involving bb to one side and all terms involving aa to the other side.\newline−3a+6b−4b=a-3a + 6b - 4b = a
  2. Combine like terms: Combine like terms to simplify the equation. −3a+2b=a-3a + 2b = a
  3. Move term involving a: Now, move the term involving a on the left side to the right side by adding 33a to both sides of the equation.\newline22b = a + 33a
  4. Combine terms involving a: Combine the terms on the right side to get a single term involving a.\newline22b = 44a
  5. Divide both sides: Divide both sides by 22 to solve for bb.\newlineb=4a2b = \frac{4a}{2}
  6. Simplify the formula: Simplify the right side to get the final formula for bb in terms of aa.\newlineb=2ab = 2a

More problems from Write equations of cosine functions using properties

Question
Find an equation for a sinusoidal function that has period 2π2\pi, amplitude 11, and contains the point (π,−1)\left(\pi, -1\right). \newlineWrite your answer in the form f(x)=Acos⁡(Bx+C)+Df(x)=A\cos(Bx+C)+D, where AA, BB, CC, and DD are real numbers.\newline f(x)=f(x)=
Get tutor helpright-arrow

Posted 7 months ago

Question
Find an equation for a sinusoidal function that has period 2π2\pi, amplitude 11, and contains the point (−π2,−1)\left(-\frac{\pi}{2},-1\right). Write your answer in the form f(x)=Asin⁡(Bx+C)+Df(x) = A\sin(Bx + C) + D, where AA, BB, CC, and DD are real numbers. `f(x)` =______
Get tutor helpright-arrow

Posted 9 months ago

Question
Find an equation for a sinusoidal function that has period 2π2\pi, amplitude 11, and contains the point (0,−1)(0,-1). \newlineWrite your answer in the form f(x)=Acos⁡(Bx+C)+Df(x) = A\cos(Bx + C) + D, where AA, BB, CC, and DD are real numbers. \newlinef(x)=f(x) = _____
Get tutor helpright-arrow

Posted 8 months ago

Question
Franklin Middle School is having a canned food drive. In Mr. Lao's class, Mr. Lao brought 1010 cans of food, and each student brought 22 cans. In Ms. Roger's class, Ms. Rogers did not bring any cans, but each student brought 33 cans of food. Write two equations in slope-intercept form to represent the number of cans of food for each class yy in terms of the number of students xx.
Get tutor helpright-arrow

Posted 8 months ago

Question
Franklin Middle School is having a canned food drive. In Mr. Lao's class, Mr. Lao brought 1010 cans of food, and each student brought 22 cans. In Ms. Roger's class, Ms. Rogers did not bring any cans, but each student brought 33 cans of food. Write two equations in slope-intercept form to represent the number of cans of food for each class yy in terms of the number of students xx.
Get tutor helpright-arrow

Posted 8 months ago

Question
Kris is wrapping Christmas lights around the railing that runs around three sides of his square porch If one side of his porch is 1212 feet long, and each strand of his Christmes lights will wrap around a 7070-inch length of the railing, what is the minimum number of strands Kris needs to completely wrap the porch railing?\newlineA) 66\newlineB) 77\newlineC) 88\newlineD) 99
Get tutor helpright-arrow

Posted 8 months ago

Question
Kris is wrapping Christmas lights around the railing that runs around three sides of his square porch If one side of his porch is 1212 feet long, and each strand of his Christmas lights will wrap around a 7070-inch length of the railing, what is the minimum number of strands Kris needs to completely wrap the porch railing?\newlineA) 66\newlineB) 77\newlineC) 88\newlineD) 99
Get tutor helpright-arrow

Posted 8 months ago

Question
For a given input value b b , the function f f outputs a value a a to satisfy the following equation.\newline4a+7b=−52 4a+7b=-52 \newlineWrite a formula for f(b) f(b) in terms of b b .\newlinef(b)=□ f(b)=\square
Get tutor helpright-arrow

Posted 6 months ago

Question
For a given input value xx, the function gg outputs a value yy to satisfy the following equation.\newline−4x−6=−5y+2-4x-6=-5y+2\newlineWrite a formula for g(x)g(x) in terms of xx.\newlineg(x)=□g(x)=\square
Get tutor helpright-arrow

Posted 10 months ago

Question
You pick a card at random, put it back, and then pick another card at random.\newline44\newline55\newline66\newline77\newlineWhat is the probability of picking a number greater than 55 and then picking a 44 ?\newlineWrite your answer as a percentage.
Get tutor helpright-arrow

Posted 1 month ago

View all (68)

Related topics

Algebra - Order of OperationsAlgebra - Distributive Property`X` and `Y` AxesGeometry - Scalene TriangleCommon MultipleGeometry - Quadrant