Resourcesdown chevron
Testimonials
Plans
Sign in
Sign up
Resourcesright arrow
Testimonials
Plans
Bytelearn - cat image with glassesAI tutor

Welcome to Bytelearn!

Let’s check out your problem:

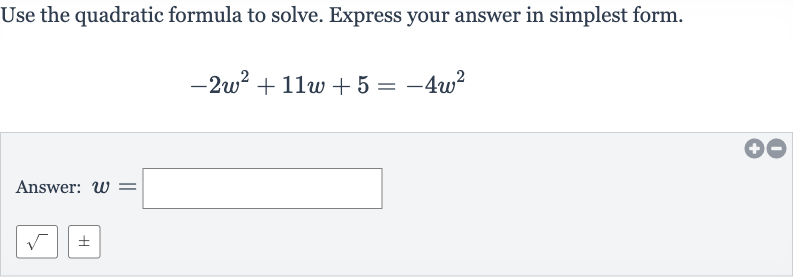
Use the quadratic formula to solve. Express your answer in simplest form. -2w^(2)+11 w+5=-4w^(2) Answer: w=

Use the quadratic formula to solve. Express your answer in simplest form.\newline−2w2+11w+5=−4w2 -2 w^{2}+11 w+5=-4 w^{2} \newlineAnswer: w= w=

View step-by-step helpView step-by-step help
Homeright chevron icon
Math Problemsright chevron icon
Algebra 2right chevron icon
Composition of linear and quadratic functions: find an equationright chevron icon

Full solution

Q. Use the quadratic formula to solve. Express your answer in simplest form.\newline−2w2+11w+5=−4w2 -2 w^{2}+11 w+5=-4 w^{2} \newlineAnswer: w= w=
  1. Simplify the equation: First, we need to simplify the equation by moving all terms to one side to get a standard quadratic equation form ax2+bx+c=0ax^2 + bx + c = 0.−2w2+11w+5=−4w2-2w^2 + 11w + 5 = -4w^2 Add 4w24w^2 to both sides to combine like terms.−2w2+4w2+11w+5=0-2w^2 + 4w^2 + 11w + 5 = 02w2+11w+5=02w^2 + 11w + 5 = 0
  2. Apply quadratic formula: Now that we have the quadratic equation in standard form, we can apply the quadratic formula to find the values of ww. The quadratic formula is given by w=−b±b2−4ac2aw = \frac{-b \pm \sqrt{b^2 - 4ac}}{2a}, where aa, bb, and cc are the coefficients from the quadratic equation ax2+bx+c=0ax^2 + bx + c = 0. In our equation, a=2a = 2, b=11b = 11, and c=5c = 5.
  3. Calculate discriminant: Next, we calculate the discriminant b2−4acb^2 - 4ac which is part of the quadratic formula.\newlineDiscriminant = b2−4acb^2 - 4ac\newlineDiscriminant = 112−4(2)(5)11^2 - 4(2)(5)\newlineDiscriminant = 121−40121 - 40\newlineDiscriminant = 8181
  4. Use quadratic formula: Since the discriminant is positive, we will have two real solutions. Now we can use the quadratic formula to find the values of ww.w=−b±Discriminant2aw = \frac{-b \pm \sqrt{\text{Discriminant}}}{2a}w=−11±812×2w = \frac{-11 \pm \sqrt{81}}{2 \times 2}w=−11±94w = \frac{-11 \pm 9}{4}
  5. Solve for w: We will now solve for w using the two possible values for the square root.\newlineFirst solution:\newlinew=(−11+9)/4w = (-11 + 9) / 4\newlinew=−2/4w = -2 / 4\newlinew=−1/2w = -1/2\newlineSecond solution:\newlinew=(−11−9)/4w = (-11 - 9) / 4\newlinew=−20/4w = -20 / 4\newlinew=−5w = -5

More problems from Composition of linear and quadratic functions: find an equation

Question
Find the inverse of the function. y=x+3 y = x + 3 Write your answer in the form ax+b ax + b . Simplify any fractions. y= y = _____
Get tutor helpright-arrow

Posted 5 months ago

Question
Find (r∘s)(x)(r \circ s)(x).\newliner(x) = 33x\newlines(x) = 22x + 55\newlineWrite your answer as a polynomial in simplest form.\newline(r∘s)(x)=(r \circ s)(x) = ____
Get tutor helpright-arrow

Posted 7 months ago

Question
What is (f+g)(x)(f + g)(x)?\newlinef(x)=−3xf(x) = -3x\newlineg(x)=3x+1g(x) = 3x + 1\newline\newlineWrite your answer as a polynomial or a rational function in simplest form.\newline‾\underline{\hspace{3cm}}\newline
Get tutor helpright-arrow

Posted 7 months ago

Question
What is (f∗g)(x)(f * g)(x)?\newlinef(x)=−x+4f(x) = -x + 4\newlineg(x)=4xg(x) = 4x\newline\newlineWrite your answer as a polynomial or a rational function in simplest form.\newline‾\underline{\hspace{3em}}\newline
Get tutor helpright-arrow

Posted 7 months ago

Question
What is the domain of (fg)(x)\left(\frac{f}{g}\right)(x)?\newlinef(x)=2xf(x) = 2x\newlineg(x)=−5x+3g(x) = -5x + 3\newlineChoices:\newline[A]All real numbers[\text{A]All real numbers}\newline[B]All real numbers except 35[\text{B]All real numbers except } \frac{3}{5}\newline[C]All real numbers except −35[\text{C]All real numbers except } -\frac{3}{5}\newline[D]All real numbers except 53[\text{D]All real numbers except } \frac{5}{3}
Get tutor helpright-arrow

Posted 9 months ago

Question
Find the inverse of the function.\newliney = −3x-3x \newlineWrite your answer in the form ax+bax + b. Simplify any fractions.\newliney = ______
Get tutor helpright-arrow

Posted 7 months ago

Question
Find (f∘g)(0)(f \circ g)(0).\newlinef(x)=6xf(x) = 6x\newlineg(x)=x2+4xg(x) = x^2 + 4x\newline(f∘g)(0)=(f \circ g)(0) = ____
Get tutor helpright-arrow

Posted 7 months ago

Question
Find (f∘g)(x)(f \circ g)(x).\newlinef(x)=x2+5f(x) = x^2 + 5\newlineg(x)=4xg(x) = 4x\newlineWrite your answer as a polynomial in simplest form.\newline(f∘g)(x)=(f \circ g)(x) = ______
Get tutor helpright-arrow

Posted 7 months ago

Question
Is f(x) f(x) the inverse function of g(x) g(x) ?\newlinef(x)=x f(x) = x \newlineg(x)=−4x g(x) = -4x \newlineChoices:\newline[yes] \text{[yes]} \newline[no] \text{[no]}
Get tutor helpright-arrow

Posted 7 months ago

Question
What is (f∗g)(x)(f * g)(x)? f(x)=3x2+9xf(x) = 3x^2 + 9x g(x)=3xg(x) = 3x Write your answer as a polynomial or a rational function in simplest form. ______
Get tutor helpright-arrow

Posted 5 months ago

View all (99)

Related topics

Algebra - Order of OperationsAlgebra - Distributive Property`X` and `Y` AxesGeometry - Scalene TriangleCommon MultipleGeometry - Quadrant