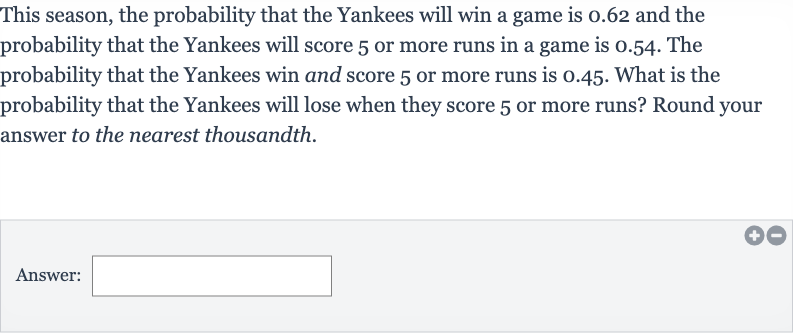
This season, the probability that the Yankees will win a game is 0.62 and the probability that the Yankees will score 5 or more runs in a game is 0.54 . The probability that the Yankees win and score 5 or more runs is 0.45 . What is the probability that the Yankees will lose when they score 5 or more runs? Round your answer to the nearest thousandth. Answer:

Question

Bytelearn · Accepted Answer

Events Denotation: Let's denote the events as follows: W: The Yankees win a game. R: The Yankees score 5 or more runs in a game. We are given the following probabilities: P(W) = 0.62 P(R) = 0.54 P(W and R) = 0.45Find Probability of Not Winning: We need to find the probability that the Yankees will lose when they score 5 or more runs. This can be represented as P(R and not W), which is the probability of scoring 5 or more runs and not winning. To find this, we first need to find the probability of not winning, which is P(not W). P(not W) = 1 - P(W) P(not W) = 1 - 0.62 P(not W) = 0.38Check Independence of Events: Now, we can use the Multiplication Rule of Probability for independent events to find P(R and not W). However, we need to check if R and not W are independent. Since we are given P(W and R), we can check for independence by comparing P(W and R) with P(W) * P(R). If they are equal, the events are independent; if not, they are dependent. Let's check: P(W) * P(R) = 0.62 * 0.54 P(W) * P(R) = 0.3348 Since P(W and R) = 0.45, which is not equal to 0.3348, the events W and R are dependent.Calculate Probability of R and not W: Since W and R are dependent, we cannot simply multiply P(R) and P(not W) to find P(R and not W). Instead, we need to use the given probabilities to find P(R and not W) by subtracting P(W and R) from P(R). P(R and not W) = P(R) - P(W and R) P(R and not W) = 0.54 - 0.45 P(R and not W) = 0.09Round Final Probability: Now that we have P(R and not W), we can round this probability to the nearest thousandth as requested. P(R and not W) ≈ 0.090

Full solution

More problems from Find probabilities using the addition rule

Related topics