Resourcesdown chevron
Testimonials
Plans
Sign in
Sign up
Resourcesright arrow
Testimonials
Plans
Bytelearn - cat image with glassesAI tutor

Welcome to Bytelearn!

Let’s check out your problem:

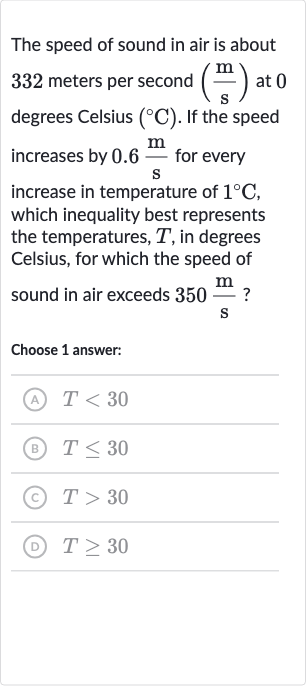
The speed of sound in air is about 332 meters per second ((m)/(s)) at 0 degrees Celsius (^(@)C). If the speed increases by 0.6(m)/(s) for every increase in temperature of 1^(@)C, which inequality best represents the temperatures, T, in degrees Celsius, for which the speed of sound in air exceeds 350(m)/(s) ? Choose 1 answer: (A) T < 30 (B) T <= 30 (C) T > 30 (D) T >= 30

The speed of sound in air is about 332ms332\frac{m}{s} at 0∘C0^\circ C. If the speed increases by 0.6ms0.6\frac{m}{s} for every increase in temperature of 1∘C1^\circ C, which inequality best represents the temperatures, TT, in degrees Celsius, for which the speed of sound in air exceeds 350ms350\frac{m}{s} ?\newlineChoose 11 answer:\newline(A) T < 30\newline(B) T≤30T \leq 30\newline(C) T > 30\newline(D) T≥30T \geq 30

View step-by-step helpView step-by-step help
Homeright chevron icon
Math Problemsright chevron icon
Algebra 1right chevron icon
Write two-variable inequalities: word problemsright chevron icon

Full solution

Q. The speed of sound in air is about 332ms332\frac{m}{s} at 0∘C0^\circ C. If the speed increases by 0.6ms0.6\frac{m}{s} for every increase in temperature of 1∘C1^\circ C, which inequality best represents the temperatures, TT, in degrees Celsius, for which the speed of sound in air exceeds 350ms350\frac{m}{s} ?\newlineChoose 11 answer:\newline(A) T<30T < 30\newline(B) T≤30T \leq 30\newline(C) T>30T > 30\newline(D) T≥30T \geq 30
  1. Given Information: We are given that the speed of sound in air at 00 degrees Celsius is 332332 meters per second. We are also told that the speed increases by 0.60.6 meters per second for every 11 degree Celsius increase in temperature. We need to find the temperature at which the speed of sound exceeds 350350 meters per second. Let's denote the temperature in degrees Celsius as TT.
  2. Equation Setup: First, we need to set up an equation that relates the speed of sound to the temperature. The speed of sound at any temperature TT can be represented as 332+0.6T332 + 0.6T, where 332332 is the speed of sound at 00 degrees Celsius and 0.6T0.6T is the increase in speed for TT degrees above 00 degrees Celsius.
  3. Inequality Setup: Next, we want to find the value of TT for which the speed of sound exceeds 350350 meters per second. So, we set up the inequality 332 + 0.6T > 350.
  4. Isolate T: Now, we solve the inequality for T. Subtract 332332 from both sides to isolate the term with TT on one side of the inequality: 0.6T > 350 - 332.
  5. Divide by 00.66: Perform the subtraction on the right side of the inequality: 0.6T > 18.
  6. Calculate T: Finally, divide both sides of the inequality by 0.60.6 to solve for TT: T > \frac{18}{0.6}.
  7. Calculate T: Finally, divide both sides of the inequality by 0.60.6 to solve for TT: T > \frac{18}{0.6}.Calculate the value of TT: T > 30.

More problems from Write two-variable inequalities: word problems

Question
Write the following inequality in slope-intercept form.\newline9x+y≥−179x+y \geq -17\newlineWrite your answer with yy first, followed by an inequality symbol. Use integers, proper fractions, and improper fractions in simplest form. \newline______
Get tutor helpright-arrow

Posted 5 months ago

Question
Does (2, 1)(2,\,1) make the inequality 2x+15y<202x + 15y < 20 true?\newlineChoices:\newlineyes\newlineno
Get tutor helpright-arrow

Posted 5 months ago

Question
Jack is fixing up his home and must spend less than $4,800\$4,800 to hire carpenters and painters. Carpenters charge $60\$60 per hour and painters charge $32\$32 per hour. \newlineSelect the inequality in standard form that describes this situation. Use the given numbers and the following variables.\newlinex=x = the number of hours of carpentry\newliney=y = the number of hours of painting\newlineChoices:\newline(A) 32x+60y<4,80032x + 60y < 4,800\newline(B) 60x+32y<4,80060x + 32y < 4,800 \newline(C) 32x−60y<4,80032x - 60y < 4,800 \newline(D) 60x−32y<4,80060x - 32y < 4,800
Get tutor helpright-arrow

Posted 5 months ago

Question
Is (2, 10)(2,\, 10) a solution to this system of inequalities?\newliney≥x+10y \geq x + 10\newliney≤9x+5y \leq 9x + 5\newlineChoices:\newline(A) yes\newline(B) no
Get tutor helpright-arrow

Posted 5 months ago

Question
Write the following inequality in slope-intercept form. \newline4x−2y≤84x - 2y \leq 8 \newline Write your answer with yy first, followed by an inequality symbol. Use integers, proper fractions, and improper fractions in simplest form.
Get tutor helpright-arrow

Posted 9 months ago

Question
Write the following inequality in slope-intercept form. \newline−3x+5y>15-3x + 5y > 15 \newline Write your answer with yy first, followed by an inequality symbol. Use integers, proper fractions, and improper fractions in simplest form.
Get tutor helpright-arrow

Posted 9 months ago

Question
Write the following inequality in slope-intercept form. \newline2x+3y<−62x + 3y < -6 \newline Write your answer with yy first, followed by an inequality symbol. Use integers, proper fractions, and improper fractions in simplest form.
Get tutor helpright-arrow

Posted 9 months ago

Question
Write the following inequality in slope-intercept form. \newline−7x−y≥21-7x - y \geq 21 \newline Write your answer with yy first, followed by an inequality symbol. Use integers, proper fractions, and improper fractions in simplest form.
Get tutor helpright-arrow

Posted 8 months ago

Question
Write the following inequality in slope-intercept form. \newline5x−4y>−205x - 4y > -20 \newline Write your answer with yy first, followed by an inequality symbol. Use integers, proper fractions, and improper fractions in simplest form.
Get tutor helpright-arrow

Posted 9 months ago

Question
Miyoko's goal is to earn more than (950(950 this week. She earns )250)250 for every day she works as a cryptographer, and earns $180\$180 for every day she works as a geologist.\newlineWrite an inequality that represents the number of days Miyoko should work as a cryptographer CC and as a geologist GG to achieve her goal.
Get tutor helpright-arrow

Posted 10 months ago

View all (97)

Related topics

Algebra - Order of OperationsAlgebra - Distributive Property`X` and `Y` AxesGeometry - Scalene TriangleCommon MultipleGeometry - Quadrant