Resources

Resources

Bytelearn - cat image with glasses

AI tutor

Welcome to Bytelearn!

Let’s check out your problem:

The graph of a sinusoidal function intersects its midline at (0,5) and then has a maximum point at (pi,6).
Write the formula of the function, where x is entered in radians.

f(x)=◻

The graph of a sinusoidal function intersects its midline at $(0,5)$ and then has a maximum point at $(\pi,6)$ . $\newline$ Write the formula of the function, where $x$ is entered in radians. $\newline$ $f(x)=$ $\square$

View step-by-step help

View step-by-step help

Write equations of sine and cosine functions using properties

Full solution

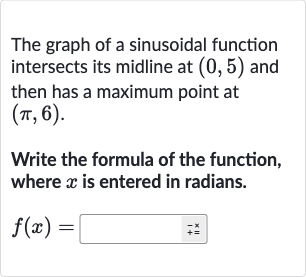
Q. The graph of a sinusoidal function intersects its midline at

(0,5)

and then has a maximum point at

(\pi,6)

.

\newline

Write the formula of the function, where

x

is entered in radians.

\newline

f(x)=

\square

Amplitude calculation: The amplitude is the distance from the midline to the maximum, so it's $6 - 5 = 1$ .
Phase shift determination: Since the graph intersects the midline at $(0,5)$ , the phase shift is $0$ .
Period calculation: The function has a maximum at $\pi$ , which means the period is $2\pi$ , so $B = 1$ .
Midline identification: The midline is the $D$ value in the equation, which is $5$ .
Function equation formation: Putting it all together, we get $f(x) = A\cos(Bx + C) + D$ , where $A = 1$ , $B = 1$ , $C = 0$ , and $D = 5$ .
Final function equation: So the equation of the function is $f(x) = \cos(x) + 5$ .

More problems from Write equations of sine and cosine functions using properties

Question

Find an equation for a sinusoidal function that has period

2\pi

1

, and contains the point

\left(\pi, -1\right)

\newline

Write your answer in the form

f(x)=A\cos(Bx+C)+D

A

B

C

D

are real numbers.

\newline

f(x)=

Posted 1 month ago

Question

Find an equation for a sinusoidal function that has period

2\pi

1

, and contains the point

\left(-\frac{\pi}{2},-1\right)

. Write your answer in the form

f(x) = A\sin(Bx + C) + D

A

B

C

D

are real numbers. `f(x)` =______

Posted 2 months ago

Question

Find an equation for a sinusoidal function that has period

2\pi

1

, and contains the point

(0,-1)

\newline

Write your answer in the form

f(x) = A\cos(Bx + C) + D

A

B

C

D

are real numbers.

\newline

f(x) =

Posted 2 months ago

Question

Franklin Middle School is having a canned food drive. In Mr. Lao's class, Mr. Lao brought

10

cans of food, and each student brought

2

cans. In Ms. Roger's class, Ms. Rogers did not bring any cans, but each student brought

3

cans of food. Write two equations in slope-intercept form to represent the number of cans of food for each class

y

in terms of the number of students

x

Posted 2 months ago

Question

Franklin Middle School is having a canned food drive. In Mr. Lao's class, Mr. Lao brought

10

cans of food, and each student brought

2

cans. In Ms. Roger's class, Ms. Rogers did not bring any cans, but each student brought

3

cans of food. Write two equations in slope-intercept form to represent the number of cans of food for each class

y

in terms of the number of students

x

Posted 2 months ago

Question

Kris is wrapping Christmas lights around the railing that runs around three sides of his square porch If one side of his porch is

12

feet long, and each strand of his Christmes lights will wrap around a

70

-inch length of the railing, what is the minimum number of strands Kris needs to completely wrap the porch railing?

\newline

6

\newline

7

\newline

8

\newline

9

Posted 2 months ago

Question

Kris is wrapping Christmas lights around the railing that runs around three sides of his square porch If one side of his porch is

12

feet long, and each strand of his Christmas lights will wrap around a

70

-inch length of the railing, what is the minimum number of strands Kris needs to completely wrap the porch railing?

\newline

6

\newline

7

\newline

8

\newline

9

Posted 2 months ago

Question

For a given input value

b

f

outputs a value

a

to satisfy the following equation.

\newline

4a+7b=-52

\newline

Write a formula for

f(b)

b

\newline

f(b)=\square

Posted 2 months ago

Question

For a given input value

x

g

outputs a value

y

to satisfy the following equation.

\newline

-4x-6=-5y+2

\newline

Write a formula for

g(x)

x

\newline

g(x)=\square

Posted 4 months ago

Question

For a given input value

a

f

outputs a value

b

to satisfy the following equation.

\newline

-3a+6b=a+4b

\newline

Write a formula for

f(a)

a

\newline

f(a)=\square

Posted 4 months ago