Resources

Resources

Bytelearn - cat image with glasses

AI tutor

Welcome to Bytelearn!

Let’s check out your problem:

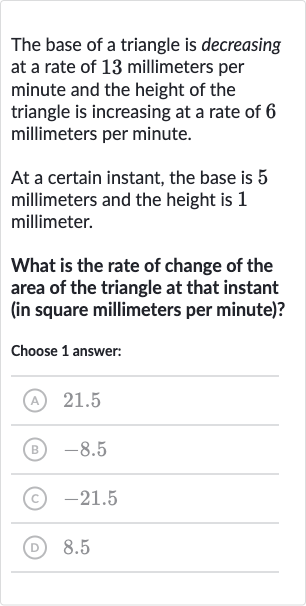
The base of a triangle is decreasing at a rate of 13 millimeters per minute and the height of the triangle is increasing at a rate of 6 millimeters per minute.
At a certain instant, the base is 5 millimeters and the height is 1 millimeter.
What is the rate of change of the area of the triangle at that instant (in square millimeters per minute)?
Choose 1 answer:
(A) 21.5
(B) -8.5
(C) -21.5
(D) 8.5

The base of a triangle is decreasing at a rate of $13$ millimeters per minute and the height of the triangle is increasing at a rate of $6$ millimeters per minute. $\newline$ At a certain instant, the base is $5$ millimeters and the height is $1$ millimeter. $\newline$ What is the rate of change of the area of the triangle at that instant (in square millimeters per minute)? $\newline$ Choose $1$ answer: $\newline$ (A) $21$ . $5$ $\newline$ (B) $-8$ . $5$ $\newline$ (C) $-21$ . $5$ $\newline$ (D) $8$ . $5$

View step-by-step help

View step-by-step help

Calculate unit rates with fractions

Full solution

Q. The base of a triangle is decreasing at a rate of

13

millimeters per minute and the height of the triangle is increasing at a rate of

6

millimeters per minute.

\newline

At a certain instant, the base is

5

millimeters and the height is

1

millimeter.

\newline

What is the rate of change of the area of the triangle at that instant (in square millimeters per minute)?

\newline

Choose

1

answer:

\newline

(A)

21

.

5

\newline

(B)

-8

.

5

\newline

(C)

-21

.

5

\newline

(D)

8

.

5

Calculate Initial Area: Now, let's calculate the initial area of the triangle with the given base and height. $\newline$ Area = $(5 \, \text{mm} \times 1 \, \text{mm}) / 2 = \frac{5}{2} \, \text{mm}^2 = 2.5 \, \text{mm}^2$ .
Find Rate of Change: To find the rate of change of the area, we'll use the formula for the derivative of the area with respect to time, $\frac{dA}{dt} = \frac{1}{2} \times (\frac{db}{dt} \times h + b \times \frac{dh}{dt})$ , where $\frac{db}{dt}$ is the rate of change of the base and $\frac{dh}{dt}$ is the rate of change of the height.
Plug in Values: We know $\frac{db}{dt} = -13 \, \text{mm/min}$ (since the base is decreasing) and $\frac{dh}{dt} = 6 \, \text{mm/min}$ (since the height is increasing). Let's plug these values into the formula. $\frac{dA}{dt} = \frac{1}{2} \times (-13 \, \text{mm/min} \times 1 \, \text{mm} + 5 \, \text{mm} \times 6 \, \text{mm/min})$ .
Perform Calculation: Now, let's do the calculation. $\newline$ $\frac{dA}{dt} = \frac{1}{2} * (-13 \, \text{mm}^2/\text{min} + 30 \, \text{mm}^2/\text{min}) = \frac{1}{2} * 17 \, \text{mm}^2/\text{min} = 8.5 \, \text{mm}^2/\text{min}$ .

More problems from Calculate unit rates with fractions

Question

What is the period of

\newline

y=\cos (-4 \pi x+3)-7 ?

\newline

Give an exact value.

\newline

Posted 3 months ago

Question

Mandy works construction. She knows that a

5

meter long metal bar has a mass of

40 \mathrm{~kg}

. Mandy wants to figure out the mass

(w)

of a bar made out of the same metal that is

3

meters long and the same thickness.

\newline

What is the mass of the shorter bar?

\newline

\mathrm{kg}

Posted 3 months ago

Question

21

6

minutes. She wants to know how many minutes

(m)

it would take her to eat

35

hot dogs if she can keep up the same pace.

\newline

How many minutes would Mika need to eat

35

\newline

Posted 3 months ago

Question

Elton is a candle maker. Each

15 \mathrm{~cm}

long candle he makes burns evenly for

6

\newline

If Elton makes a

45 \mathrm{~cm}

long candle, how long would it burn?

\newline

Posted 3 months ago

Question

Harvey the wonder hamster can run

3 \frac{1}{6} \mathrm{~km}

\frac{1}{4}

hour. Harvey runs at a constant rate.

\newline

Find his average speed in kilometers per hour.

\newline

kilometers per hour

Posted 3 months ago

Question

Aliyyah is playing a game using dice. Each player takes turns rolling and adding their roll to their score.

\newline

Aliyyah started with

-19

points and ended with

7

\newline

How many points did Aliyyah score during the game?

\newline

Posted 3 months ago

Question

A scale on a blue print drawing of a house shows that

6

centimeters represents

3

\newline

What number of centimeters on the blue print represents an actual distance of

27

\newline

Posted 3 months ago

Question

Reem is a test driver for an automobile company. The following formula gives the total distance,

d

, in feet that Reem drove a luxury car in the first

t

seconds after idling at a speed of

0

miles per hour, up to the time when she passed a particular safety cone.

\newline

d=15.69 t^{2}

\newline

Compared to the time it took Reem to pass the safety cone, how long did it take to pass a sensor that was

\frac{4}{9}

of the distance from the start?

\newline

1

\newline

\frac{16}{81}

of the time it required to pass the cone

\newline

\frac{4}{9}

of the time it required to pass the cone

\newline

\frac{2}{3}

of the time it required to pass the cone

\newline

\frac{9}{4}

of the time it required to pass the cone

Posted 3 months ago

Question

\newline

4

\newline

\newline

\newline

\newline

\newline

stera be chanchat

\newline

\newline

\newline

1

2

\newline

What is the image point of

\newline

(-6,-1)

after the transformation

\newline

R_{180}@r_{y=-x}

\newline

(1)

\newline

Posted 2 months ago

Question

VKB

VK=20.6\,\text{centimeters (cm)}, VB=21.5\,\text{cm}, BK=31.9\,\text{cm}

VR=17\,\text{cm}

. Which is the area of

\triangle VKB

\newline

271.15\,\text{cm}^2

\newline

221.45\,\text{cm}^2

\newline

175.1\,\text{cm}^2

\newline

542.3\,\text{cm}^2

\newline

\newline

\hat{\text{TOp}}

Posted 2 months ago