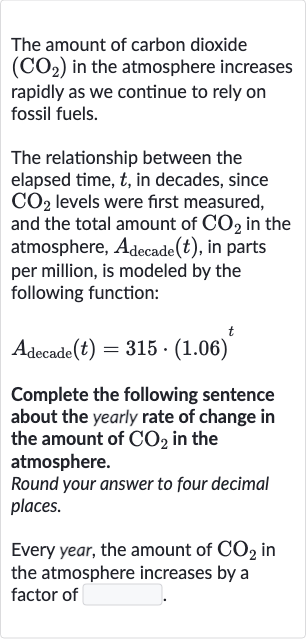
The amount of carbon dioxide $\left(\mathrm{CO}_{2}\right)$ in the atmosphere increases rapidly as we continue to rely on fossil fuels. $\newline$ The relationship between the elapsed time, $t$ , in decades, since $\mathrm{CO}_{2}$ levels were first measured, and the total amount of $\mathrm{CO}_{2}$ in the atmosphere, $A_{\text {decade }}(t)$ , in parts per million, is modeled by the following function: $\newline$ $A_{\text {decade }}(t)=315 \cdot(1.06)^{t}$ $\newline$ Complete the following sentence about the yearly rate of change in the amount of $\mathrm{CO}_{2}$ in the atmosphere. $\newline$ Round your answer to four decimal places. $\newline$ Every year, the amount of $\mathrm{CO}_{2}$ in the atmosphere increases by a factor of

Full solution

Q. The amount of carbon dioxide

\left(\mathrm{CO}_{2}\right)

in the atmosphere increases rapidly as we continue to rely on fossil fuels.

\newline

The relationship between the elapsed time,

t

, in decades, since

\mathrm{CO}_{2}

levels were first measured, and the total amount of

\mathrm{CO}_{2}

in the atmosphere,

A_{\text {decade }}(t)

, in parts per million, is modeled by the following function:

\newline

A_{\text {decade }}(t)=315 \cdot(1.06)^{t}

\newline

Complete the following sentence about the yearly rate of change in the amount of

\mathrm{CO}_{2}

in the atmosphere.

\newline

Round your answer to four decimal places.

\newline

Every year, the amount of

\mathrm{CO}_{2}

in the atmosphere increases by a factor of

Understand function representation: Understand the given function and what it represents. $\newline$ The function $A_{\text{decade}}(t)=315\times(1.06)^{t}$ models the total amount of $\text{CO}_2$ in the atmosphere in parts per million as a function of time in decades since $\text{CO}_2$ levels were first measured. The base amount of $\text{CO}_2$ is $315\,\text{ppm}$ , and it increases by a factor of $1.06$ every decade.
Determine yearly rate change: Determine the yearly rate of change. $\newline$ Since the function gives the amount of CO $_2$ after $t$ decades, we need to find the equivalent yearly rate of change. There are $10$ years in a decade, so we need to take the $10$ th root of $1.06$ to find the yearly rate of change.
Calculate $10$ th root: Calculate the $10$ th root of $1.06$ . The $10$ th root of $1.06$ can be calculated using the formula $(1.06)^{\frac{1}{10}}$ .
Perform calculation: Perform the calculation. $\newline$ Using a calculator, we find that $(1.06)^{\frac{1}{10}}$ is approximately equal to $1.0058$ when rounded to four decimal places.
Interpret yearly increase: Interpret the result. $\newline$ The calculation shows that every year, the amount of CO extsubscript{ $2$ } in the atmosphere increases by a factor of approximately $1.0058$ .