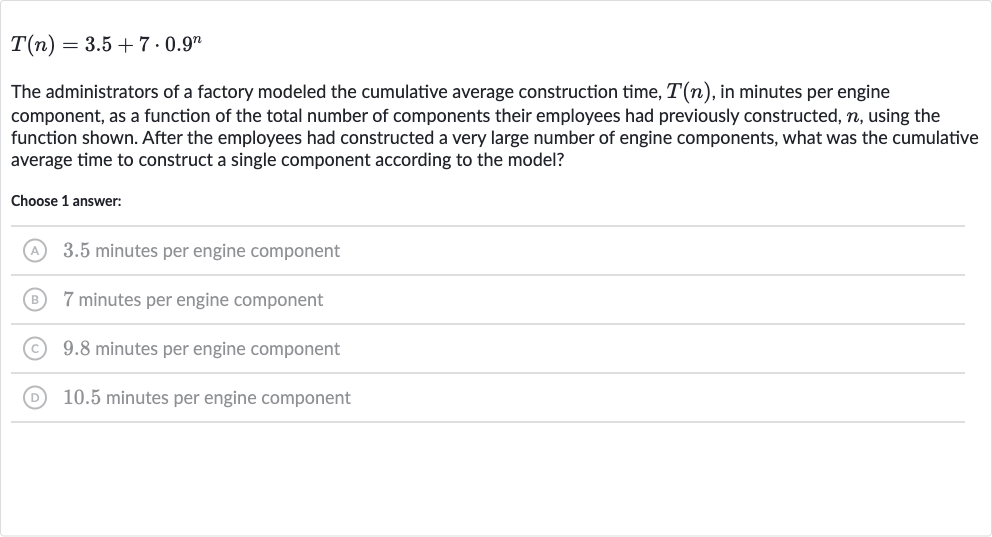
$T(n)=3.5+7 \cdot 0.9^{n}$ $\newline$ The administrators of a factory modeled the cumulative average construction time, $T(n)$ , in minutes per engine component, as a function of the total number of components their employees had previously constructed, $n$ , using the function shown. After the employees had constructed a very large number of engine components, what was the cumulative average time to construct a single component according to the model? $\newline$ Choose $1$ answer: $\newline$ (A) $3$ . $5$ minutes per engine component $\newline$ (B) $7$ minutes per engine component $\newline$ (C) $9$ . $8$ minutes per engine component $\newline$ (D) $10$ . $5$ minutes per engine component

View step-by-step help

Home

Math Problems

Algebra 1

Solve quadratic equations: word problems

Full solution

T(n)=3.5+7 \cdot 0.9^{n}

\newline

The administrators of a factory modeled the cumulative average construction time,

T(n)

, in minutes per engine component, as a function of the total number of components their employees had previously constructed,

n

, using the function shown. After the employees had constructed a very large number of engine components, what was the cumulative average time to construct a single component according to the model?

\newline

Choose

1

answer:

\newline

(A)

3

5

minutes per engine component

\newline

(B)

7

minutes per engine component

\newline

(C)

9

8

minutes per engine component

\newline

(D)

10

5

minutes per engine component

Approaching $0$ : As $n$ approaches a very large number, the term $0.9^n$ approaches $0$ because $0.9$ is less than $1$ and any number less than $1$ raised to a large power gets closer and closer to $0$ .
Function Simplification: So, the function $T(n) = 3.5 + 7 \times 0.9^n$ will approach $3.5 + 7 \times 0$ , because $0.9^n$ is almost $0$ .
Calculating Limit: Now, calculate the limit of $T(n)$ as $n$ approaches infinity: $T(n) = 3.5 + 7 \times 0 = 3.5$ .
Final Result: Therefore, the cumulative average time to construct a single engine component after constructing a very large number of components is $3.5$ minutes per engine component.