Resources

Resources

Bytelearn - cat image with glasses

AI tutor

Welcome to Bytelearn!

Let’s check out your problem:

Solve the equation
x^(2)-6x-37=0 to the nearest tenth.
Answer:
x=

Solve the equation $x^{2}-6 x-37=0$ to the nearest tenth. $\newline$ Answer: $x=$

View step-by-step help

View step-by-step help

Solve exponential equations using logarithms

Full solution

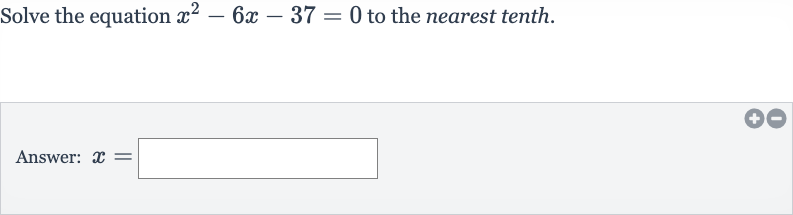
Q. Solve the equation

x^{2}-6 x-37=0

to the nearest tenth.

\newline

Answer:

x=

Identify Equation Type: Identify the type of equation. $\newline$ We have a quadratic equation in the form of $ax^2 + bx + c = 0$ , where $a = 1$ , $b = -6$ , and $c = -37$ .
Use Quadratic Formula: Use the quadratic formula to solve for $x$ . The quadratic formula is $x = \frac{-b \pm \sqrt{b^2 - 4ac}}{2a}$ . Here, $a = 1$ , $b = -6$ , and $c = -37$ .
Calculate Discriminant: Calculate the discriminant $b^2 - 4ac$ . $\newline$ Discriminant = $(-6)^2 - 4(1)(-37) = 36 + 148 = 184$ .
Calculate Solutions: Calculate the two solutions using the quadratic formula. $\newline$ $x = \frac{-(-6) \pm \sqrt{184}}{2 \cdot 1}$ $\newline$ $x = \frac{6 \pm \sqrt{184}}{2}$
Calculate First Solution: Calculate the first solution (using the + sign). $\newline$ $x = \frac{6 + \sqrt{184}}{2}$ $\newline$ $x \approx \frac{6 + 13.5647}{2}$ $\newline$ $x \approx \frac{19.5647}{2}$ $\newline$ $x \approx 9.78235$ $\newline$ Round to the nearest tenth: $x \approx 9.8$
Calculate Second Solution: Calculate the second solution (using the - sign). $\newline$ $x = \frac{6 - \sqrt{184}}{2}$ $\newline$ $x \approx \frac{6 - 13.5647}{2}$ $\newline$ $x \approx \frac{-7.5647}{2}$ $\newline$ $x \approx -3.78235$ $\newline$ Round to the nearest tenth: $x \approx -3.8$

More problems from Solve exponential equations using logarithms

Question

Solve. Write your answer as an integer or a fraction in simplest form.

\newline

5^x=1

\newline

x=

Posted 2 months ago

Question

Steven opened a savings account and deposited

\$100.00

as principal. The account earns

9\%

interest, compounded annually. What is the balance after

5

\newline

Use the formula

A = P(1 + \frac{r}{n})^{nt}

A

is the balance (final amount),

P

is the principal (starting amount),

r

is the interest rate expressed as a decimal,

n

is the number of times per year that the interest is compounded, and

t

is the time in years.

\newline

Round your answer to the nearest cent.

\newline

\$

Posted 2 months ago

Question

Use the following function rule to find

f(1)

\newline

f(x) = 12(7)^x + 8

\newline

f(1) =

Posted 1 month ago

Question

The town of Valley View conducted a census this year, which showed that it has a population of

1,800

people. Based on the census data, it is estimated that the population of Valley View will grow by

12\%

\newline

Write an exponential equation in the form

y = a(b)^x

that can model the town population,

y

x

decades after this census was taken.

\newline

Use whole numbers, decimals, or simplified fractions for the values of

a

b

\newline

y =

Posted 2 months ago

Question

Solve. Round your answer to the nearest thousandth.

\newline

7 = 9^x

\newline

x =

Posted 2 months ago

Question

Solve. Round your answer to the nearest thousandth.

\newline

7 = e^x

\newline

x =

Posted 1 month ago

Question

Solve. Simplify your answer.

\newline

\log u = 1

\newline

u =

Posted 2 months ago

Question

Solve. Simplify your answer.

\newline

7\log x = 7

\newline

x =

Posted 1 month ago

Question

g(t) = 3^t

change over the interval from

t = 3

t = 4

\newline

\newline

g(t)

3

\newline

g(t)

3

\newline

g(t)

3\%

\newline

g(t)

200\%

Posted 2 months ago

Question

f(x)

6

over every unit interval in

x

f(0) = 0

\newline

Which could be a function rule for

f(x)

\newline

\newline

f(x) = 6^x

\newline

f(x) = 6x

\newline

f(x) = \frac{1}{6^x}

\newline

f(x) = \frac{x}{6}

Posted 2 months ago