AI tutor
Full solution
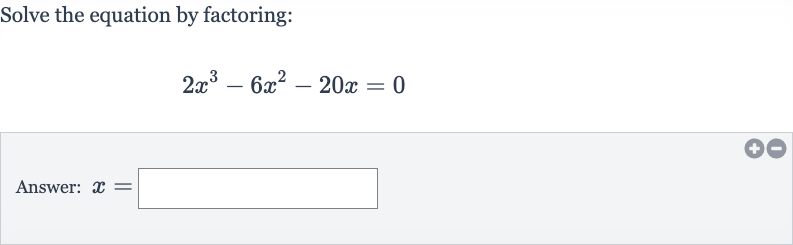
Q. Solve the equation by factoring:Answer:
- Factor out GCF: First, we need to factor out the greatest common factor (GCF) from the given cubic equation . The GCF of , , and is . So we factor out of each term.
- Factor quadratic equation: Now we need to factor the quadratic equation . We look for two numbers that multiply to and add up to . These numbers are and . So we can write the quadratic as .
- Set factor equal to zero: To find the roots of the equation, we set each factor equal to zero and solve for . First, we set the factor equal to zero:
- Set factor equal to zero: Next, we set the factor equal to zero:
- Set factor equal to zero: Finally, we set the factor equal to zero: