AI tutor
Full solution
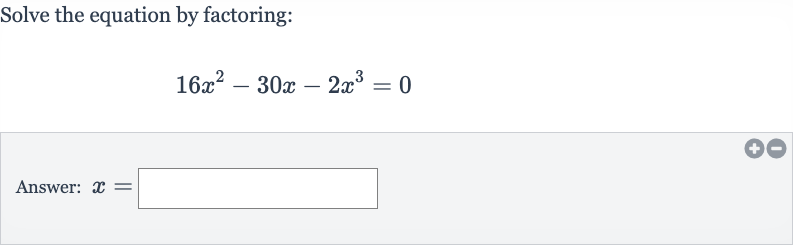
Q. Solve the equation by factoring:Answer:
- Rewrite in Standard Form: First, let's rewrite the equation in standard form by arranging the terms in descending order of their exponents.The given equation is .Rearrange the terms to get .
- Factor Out Common Factor: Next, factor out the greatest common factor, which in this case is . So, .
- Factor Quadratic Expression: Now, we need to factor the quadratic expression . To find factors of that add up to , we can use and . So, can be factored as .
- Find Roots: The factored form of the original equation is now . To find the roots, we set each factor equal to zero and solve for . , , and .
- Find Roots: The factored form of the original equation is now .To find the roots, we set each factor equal to zero and solve for ., , and .Solving each equation for gives us the roots:From , we get .From , we get .From , we get .