Resources

Resources

Bytelearn - cat image with glasses

AI tutor

Welcome to Bytelearn!

Let’s check out your problem:

Solve for
x, rounding to the nearest hundredth.

3^(x)=45
Answer:

Solve for $x$ , rounding to the nearest hundredth. $\newline$ $3^{x}=45$ $\newline$ Answer:

View step-by-step help

View step-by-step help

Solve exponential equations using logarithms

Full solution

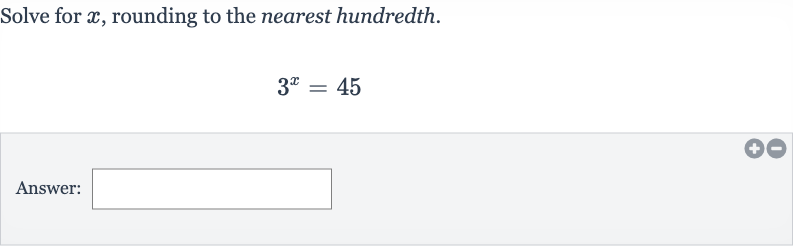
Q. Solve for

x

, rounding to the nearest hundredth.

\newline

3^{x}=45

\newline

Answer:

Write Equation: Write down the equation that needs to be solved. $\newline$ We have the equation $3^{x} = 45$ .
Apply Logarithm: Apply the logarithm to both sides of the equation to solve for $x$ . Taking the natural logarithm ( $\ln$ ) of both sides gives us $\ln(3^{x}) = \ln(45)$ .
Use Power Property: Use the power property of logarithms to simplify the left side of the equation. $\newline$ The power property of logarithms states that $\ln(a^b) = b\cdot\ln(a)$ . Applying this to our equation gives us $x\cdot\ln(3) = \ln(45)$ .
Isolate $x$ : Isolate $x$ by dividing both sides of the equation by $\ln(3)$ . This gives us $x = \frac{\ln(45)}{\ln(3)}$ .
Calculate x: Calculate the value of x using a calculator. $\newline$ $x = \frac{\ln(45)}{\ln(3)} \approx x = \frac{3.80666248977}{1.09861228867} \approx x = 3.4657359028.$
Round to Nearest: Round the calculated value of $x$ to the nearest hundredth. $\newline$ Rounding $x = 3.4657359028$ to the nearest hundredth gives us $x \approx 3.47$ .

More problems from Solve exponential equations using logarithms

Question

Solve. Write your answer as an integer or a fraction in simplest form.

\newline

5^x=1

\newline

x=

Posted 2 months ago

Question

Steven opened a savings account and deposited

\$100.00

as principal. The account earns

9\%

interest, compounded annually. What is the balance after

5

\newline

Use the formula

A = P(1 + \frac{r}{n})^{nt}

A

is the balance (final amount),

P

is the principal (starting amount),

r

is the interest rate expressed as a decimal,

n

is the number of times per year that the interest is compounded, and

t

is the time in years.

\newline

Round your answer to the nearest cent.

\newline

\$

Posted 2 months ago

Question

Use the following function rule to find

f(1)

\newline

f(x) = 12(7)^x + 8

\newline

f(1) =

Posted 1 month ago

Question

The town of Valley View conducted a census this year, which showed that it has a population of

1,800

people. Based on the census data, it is estimated that the population of Valley View will grow by

12\%

\newline

Write an exponential equation in the form

y = a(b)^x

that can model the town population,

y

x

decades after this census was taken.

\newline

Use whole numbers, decimals, or simplified fractions for the values of

a

b

\newline

y =

Posted 2 months ago

Question

Solve. Round your answer to the nearest thousandth.

\newline

7 = 9^x

\newline

x =

Posted 2 months ago

Question

Solve. Round your answer to the nearest thousandth.

\newline

7 = e^x

\newline

x =

Posted 1 month ago

Question

Solve. Simplify your answer.

\newline

\log u = 1

\newline

u =

Posted 2 months ago

Question

Solve. Simplify your answer.

\newline

7\log x = 7

\newline

x =

Posted 1 month ago

Question

g(t) = 3^t

change over the interval from

t = 3

t = 4

\newline

\newline

g(t)

3

\newline

g(t)

3

\newline

g(t)

3\%

\newline

g(t)

200\%

Posted 2 months ago

Question

f(x)

6

over every unit interval in

x

f(0) = 0

\newline

Which could be a function rule for

f(x)

\newline

\newline

f(x) = 6^x

\newline

f(x) = 6x

\newline

f(x) = \frac{1}{6^x}

\newline

f(x) = \frac{x}{6}

Posted 2 months ago