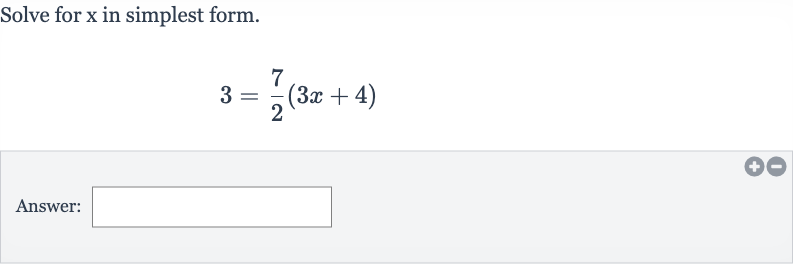Resourcesdown chevron
Testimonials
Plans
Sign in
Sign up
Resourcesright arrow
Testimonials
Plans
Bytelearn - cat image with glassesAI tutor

Welcome to Bytelearn!

Let’s check out your problem:

Solve for x in simplest form. 3=(7)/(2)(3x+4) Answer:

Solve for x \mathrm{x} in simplest form.\newline3=72(3x+4) 3=\frac{7}{2}(3 x+4) \newlineAnswer:

View step-by-step helpView step-by-step help
Homeright chevron icon
Math Problemsright chevron icon
Precalculusright chevron icon
Find the roots of factored polynomialsright chevron icon

Full solution

Q. Solve for x \mathrm{x} in simplest form.\newline3=72(3x+4) 3=\frac{7}{2}(3 x+4) \newlineAnswer:
  1. Isolate variable xx: First, we need to isolate the term with the variable xx on one side of the equation. To do this, we can multiply both sides of the equation by the reciprocal of the fraction 72\frac{7}{2}, which is 27\frac{2}{7}. This will cancel out the fraction on the right side of the equation.\newlineCalculation: 27×3=27×72(3x+4)\frac{2}{7} \times 3 = \frac{2}{7} \times \frac{7}{2}(3x + 4)
  2. Simplify left side: After multiplying both sides by (27)(\frac{2}{7}), we simplify the left side of the equation.\newlineCalculation: (27)×3=67(\frac{2}{7}) \times 3 = \frac{6}{7}
  3. Cancel fractions: On the right side, the (72)(\frac{7}{2}) and (27)(\frac{2}{7}) cancel each other out, leaving us with 3x+43x + 4.\newlineCalculation: (27)×(72)(3x+4)=3x+4(\frac{2}{7}) \times (\frac{7}{2})(3x + 4) = 3x + 4
  4. Subtract 44: Now we have a simplified equation: 67=3x+4\frac{6}{7} = 3x + 4. The next step is to subtract 44 from both sides to isolate the term with xx.\newlineCalculation: 67−4=3x+4−4\frac{6}{7} - 4 = 3x + 4 - 4
  5. Combine terms: Subtracting 44 from 67\frac{6}{7}, we need to express 44 as a fraction with a denominator of 77 to combine the terms. 44 is equivalent to 287\frac{28}{7}.\newlineCalculation: 67−287=3x\frac{6}{7} - \frac{28}{7} = 3x
  6. Subtract fractions: Now we subtract the fractions: 67−287=−227\frac{6}{7} - \frac{28}{7} = -\frac{22}{7}.\newlineCalculation: 67−287=−227\frac{6}{7} - \frac{28}{7} = -\frac{22}{7}
  7. Divide by 33: We now have the equation −227=3x-\frac{22}{7} = 3x. To solve for x, we divide both sides by 33.\newlineCalculation: (−227)/3=3x3\left(-\frac{22}{7}\right) / 3 = \frac{3x}{3}
  8. Multiply by reciprocal: Dividing −227-\frac{22}{7} by 33 is the same as multiplying by the reciprocal of 33, which is 13\frac{1}{3}.\newlineCalculation: (−227)×(13)=x(-\frac{22}{7}) \times (\frac{1}{3}) = x
  9. Final result: Multiplying −227-\frac{22}{7} by 13\frac{1}{3} gives us −2221-\frac{22}{21}.\newlineCalculation: (−227)×(13)=−2221(-\frac{22}{7}) \times (\frac{1}{3}) = -\frac{22}{21}

More problems from Find the roots of factored polynomials

Question
Find the degree of this polynomial.\newline`5b^{10} + 3b^3`\newline_______
Get tutor helpright-arrow

Posted 5 months ago

Question
Subtract.\newline(9a+5)−(2a+4)(9a + 5) - (2a + 4)\newline____
Get tutor helpright-arrow

Posted 5 months ago

Question
Find the product. Simplify your answer. \newline−3v2(v2−9)-3v^2(v^2 - 9)\newline________
Get tutor helpright-arrow

Posted 5 months ago

Question
Find the product. Simplify your answer.\newline(v−3)(4v+1)(v - 3)(4v + 1)\newline______
Get tutor helpright-arrow

Posted 5 months ago

Question
Find the square. Simplify your answer.\newline(3y+2)2(3y + 2)^2\newline______
Get tutor helpright-arrow

Posted 5 months ago

Question
Divide. If there is a remainder, include it as a simplified fraction.\newline(24t2+36t)÷6t(24t^2 + 36t) \div 6t\newline______
Get tutor helpright-arrow

Posted 5 months ago

Question
Is the function q(x)=x6−9 q(x) = x^6 - 9 even, odd, or neither?\newlineChoices:\newline[[even][odd][neither]]\text{[[even][odd][neither]]}
Get tutor helpright-arrow

Posted 9 months ago

Question
Find the product. Simplify your answer. \newline−3q2(−3q2+q)-3q^2(-3q^2 + q)\newline______
Get tutor helpright-arrow

Posted 9 months ago

Question
Find the product. Simplify your answer.\newline(r+3)(4r+2)(r + 3)(4r + 2)\newline______
Get tutor helpright-arrow

Posted 9 months ago

Question
Find the roots of the factored polynomial.\newline(x+7)(x+4)(x + 7)(x + 4)\newlineWrite your answer as a list of values separated by commas.\newlinex=x = ____
Get tutor helpright-arrow

Posted 9 months ago

View all (260)

Related topics

Algebra - Order of OperationsAlgebra - Distributive Property`X` and `Y` AxesGeometry - Scalene TriangleCommon MultipleGeometry - Quadrant