AI tutor
Full solution
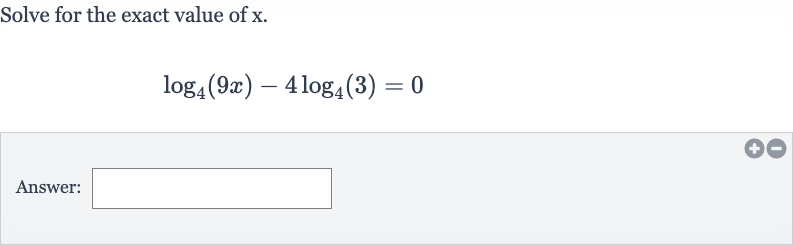
Q. Solve for the exact value of .Answer:
- Apply power rule: Apply the power rule of logarithms to simplify the term with the coefficient.The power rule of logarithms states that . Let's apply this to the term .
- Calculate value: Calculate the value of .
- Rewrite equation: Rewrite the equation using the result from Step .
- Apply quotient rule: Apply the quotient rule of logarithms to combine the logarithms.The quotient rule of logarithms states that . Let's apply this to combine the logarithms.
- Simplify fraction: Simplify the fraction inside the logarithm. So,
- Convert to exponential: Convert the logarithmic equation to an exponential equation.If , then . Let's apply this to solve for .
- Calculate value: Calculate the value of .
- Solve for x: Solve for x.