Resources

Resources

Bytelearn - cat image with glasses

AI tutor

Welcome to Bytelearn!

Let’s check out your problem:

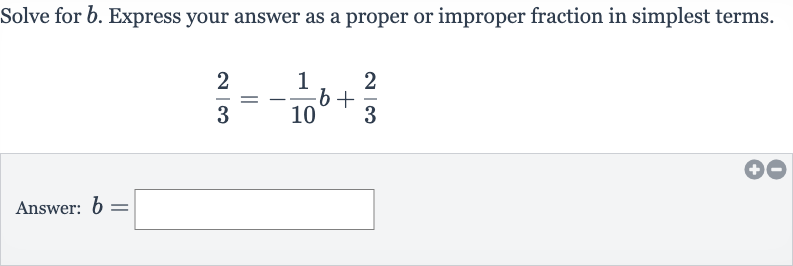
$Solve for b. Express your answer as a proper or improper fraction in simplest terms. (2)/(3)=-(1)/(10)b+(2)/(3) Answer: b=$

Solve for $b$ . Express your answer as a proper or improper fraction in simplest terms. $\newline$ $\frac{2}{3}=-\frac{1}{10} b+\frac{2}{3}$ $\newline$ Answer: $b=$

View step-by-step help

View step-by-step help

Find the roots of factored polynomials

Full solution

Q. Solve for

b

. Express your answer as a proper or improper fraction in simplest terms.

\newline

\frac{2}{3}=-\frac{1}{10} b+\frac{2}{3}

\newline

Answer:

b=

Write Equation: First, let's write down the equation we need to solve: $\newline$ rac{2}{3} = -rac{1}{10}b + rac{2}{3}
Simplify by Subtracting: We notice that $(\frac{2}{3})$ appears on both sides of the equation. To simplify, we can subtract $(\frac{2}{3})$ from both sides to eliminate it: $(\frac{2}{3}) - (\frac{2}{3}) = -(\frac{1}{10})b + (\frac{2}{3}) - (\frac{2}{3})$
Solve for $b$ : After subtracting $\frac{2}{3}$ from both sides, we get: $\newline$ $0 = -\frac{1}{10}b$
Isolate b: Now, we need to solve for b. Since $-(\frac{1}{10})b = 0$ , we can divide both sides by $-(\frac{1}{10})$ to isolate b: $\newline$ $\frac{0}{-(\frac{1}{10})} = b$
Final Answer: Dividing $0$ by any non-zero number is still $0$ , so we have: $\newline$ $b = 0$

More problems from Find the roots of factored polynomials

Question

Find the degree of this polynomial.

\newline

`5b^{10} + 3b^3`

\newline

Posted 5 months ago

Question

\newline

(9a + 5) - (2a + 4)

\newline

Posted 5 months ago

Question

Find the product. Simplify your answer.

\newline

-3v^2(v^2 - 9)

\newline

Posted 5 months ago

Question

Find the product. Simplify your answer.

\newline

(v - 3)(4v + 1)

\newline

Posted 5 months ago

Question

Find the square. Simplify your answer.

\newline

(3y + 2)^2

\newline

Posted 5 months ago

Question

Divide. If there is a remainder, include it as a simplified fraction.

\newline

(24t^2 + 36t) \div 6t

\newline

Posted 5 months ago

Question

Is the function

q(x) = x^6 - 9

even, odd, or neither?

\newline

\newline

\text{[[even][odd][neither]]}

Posted 9 months ago

Question

Find the product. Simplify your answer.

\newline

-3q^2(-3q^2 + q)

\newline

Posted 9 months ago

Question

Find the product. Simplify your answer.

\newline

(r + 3)(4r + 2)

\newline

Posted 9 months ago

Question

Find the roots of the factored polynomial.

\newline

(x + 7)(x + 4)

\newline

Write your answer as a list of values separated by commas.

\newline

x =

Posted 9 months ago

Related topics

Algebra - Order of Operations Algebra - Distributive Property `X` and `Y` Axes Geometry - Scalene Triangle Common Multiple Geometry - Quadrant