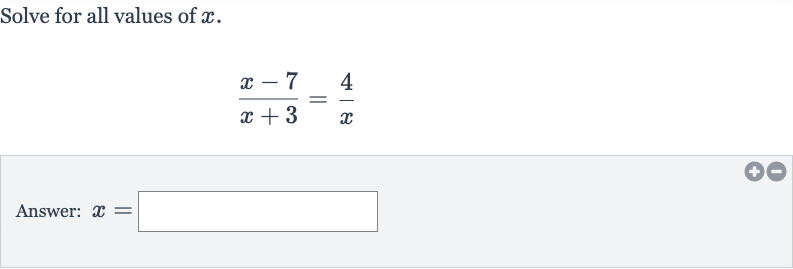
Solve for all values of x. (x-7)/(x+3)=(4)/(x) Answer: x=

Question

Solve for all values of  x.  (x-7)/(x+3)=(4)/(x) Answer:  x=

Bytelearn · Accepted Answer

Find Common Denominator: First, we need to find a common denominator to combine the fractions on both sides of the equation. The common denominator here is x(x+3).Multiply by Common Denominator: Next, we multiply both sides of the equation by the common denominator to eliminate the fractions: x(x+3) * (x-7)/(x+3) = x(x+3) * (4)/(x)Simplify Equation: Simplify the equation by canceling out the common terms on both sides: x(x-7) = 4(x+3)Distribute Terms: Now, distribute the x on the left side and the 4 on the right side: x^2 - 7x = 4x + 12Combine Like Terms: Bring all terms to one side to set the equation to zero and combine like terms: x^2 - 7x - 4x - 12 = 0 x^2 - 11x - 12 = 0Factor Quadratic Equation: We now have a quadratic equation. To solve for x, we can factor the quadratic equation: (x - 12)(x + 1) = 0Solve for x: Set each factor equal to zero and solve for x: x - 12 = 0 or x + 1 = 0 x = 12 or x = -1Check for Extraneous Solutions: We must check for extraneous solutions by plugging the values back into the original equation, because the original equation has restrictions (x cannot be 0 or -3, as those would make the denominators zero).Check x = 12: Check x = 12: (12-7)/(12+3) = 4/12 5/15 = 4/12 1/3 = 1/3 (This is true, so x = 12 is a valid solution.)Check x = -1: Check x = -1: (-1-7)/(-1+3) = 4/(-1) -8/2 = -4 -4 = -4 (This is true, so x = -1 is a valid solution.)

Solve for all values of $x$ . $\newline$ $\frac{x-7}{x+3}=\frac{4}{x}$ $\newline$ Answer: $x=$

Full solution

More problems from Find the roots of factored polynomials

Related topics

Solve for all values of x x x.\newlinex−7x+3=4x \frac{x-7}{x+3}=\frac{4}{x} x+3x−7​=x4​\newlineAnswer: x= x= x=

Solve for all values of $x$ . $\newline$ $\frac{x-7}{x+3}=\frac{4}{x}$ $\newline$ Answer: $x=$