AI tutor
Full solution
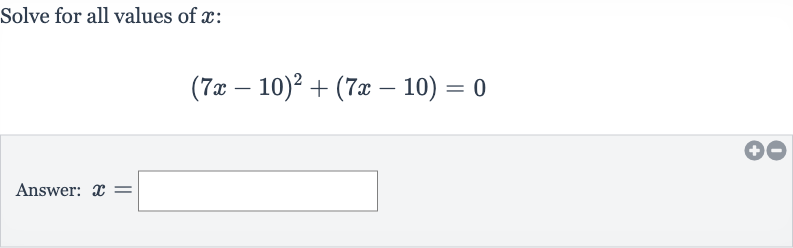
Q. Solve for all values of :Answer:
- Set up the equation: Let's set up the equation and identify it as a quadratic in the form of .
- Factor by grouping: We can factor by grouping since we have a common factor of . Let , then the equation becomes:
- Factor the equation: Now we factor the left side of the equation as .
- Simplify factors: Simplify the factors to find the roots of the equation.
- Solve for x: Set each factor equal to zero and solve for x.First factor:
- Second factor: Now solve for using the second factor.Second factor:
More problems from Solve multi-step equations with fractional coefficients
QuestionGet tutor help