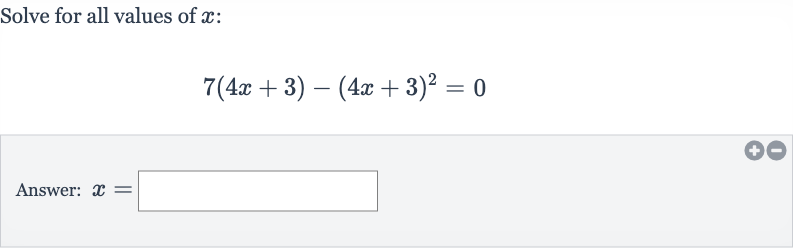AI tutor
Full solution
Q. Solve for all values of :Answer:
- Expand Equation: Expand the equation to simplify it.We have the equation . Let's first expand the squared term .
- Substitute Expanded Term: Substitute the expanded squared term back into the equation.Now we substitute the expanded back into the original equation:
- Expand and Combine Terms: Expand the remaining term and combine like terms.Next, we expand and subtract from it:So the equation becomes:
- Distribute Negative Sign: Distribute the negative sign through the second set of parentheses.We need to distribute the negative sign through the second set of parentheses:
- Combine Like Terms: Combine like terms to form a quadratic equation.Now we combine like terms:
- Factor Quadratic Equation: Factor the quadratic equation.We need to factor the quadratic equation . We can factor out a :Now we need to factor the quadratic expression in the parentheses. However, this expression does not factor nicely, so we may have made a mistake. Let's go back and check our previous steps.
More problems from Composition of linear and quadratic functions: find an equation
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help