Resources

Resources

Bytelearn - cat image with glasses

AI tutor

Welcome to Bytelearn!

Let’s check out your problem:

Solve for a positive value of
x.

log_(x)(216)=3
Answer:

Solve for a positive value of $x$ . $\newline$ $\log _{x}(216)=3$ $\newline$ Answer:

View step-by-step help

View step-by-step help

Product property of logarithms

Full solution

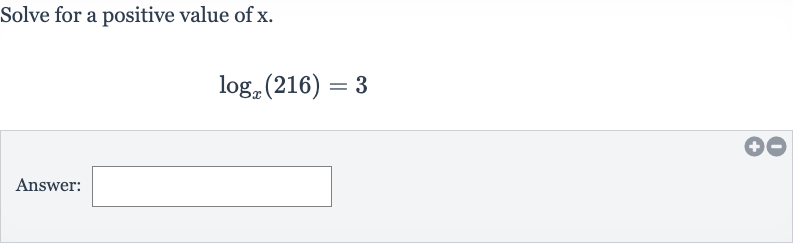
Q. Solve for a positive value of

x

.

\newline

\log _{x}(216)=3

\newline

Answer:

Understand the equation: Understand the logarithmic equation. $\newline$ The equation $\log_{x}(216) = 3$ means that $x$ raised to the power of $3$ equals $216$ .
Convert to exponential: Convert the logarithmic equation to an exponential equation. $\newline$ Using the definition of a logarithm, we can rewrite the equation as $x^3 = 216$ .
Solve for x: Solve for x by taking the cube root of both sides. $\newline$ To find $x$ , we take the cube root of $216$ , which is $x = 216^{(1/3)}$ .
Calculate cube root: Calculate the cube root of $216$ . The cube root of $216$ is $6$ , because $6^3 = 216$ .
Verify the solution: Verify the solution. $\newline$ We substitute $x = 6$ back into the original equation to check if it satisfies the equation: $\log_{6}(216) = 3$ . $\newline$ Since $6^3 = 216$ , the equation holds true, confirming that $x = 6$ is the correct solution.

More problems from Product property of logarithms

Question

Solve. Write your answer as an integer or a fraction in simplest form.

\newline

5^x=1

\newline

x=

Posted 2 months ago

Question

Steven opened a savings account and deposited

\$100.00

as principal. The account earns

9\%

interest, compounded annually. What is the balance after

5

\newline

Use the formula

A = P(1 + \frac{r}{n})^{nt}

A

is the balance (final amount),

P

is the principal (starting amount),

r

is the interest rate expressed as a decimal,

n

is the number of times per year that the interest is compounded, and

t

is the time in years.

\newline

Round your answer to the nearest cent.

\newline

\$

Posted 2 months ago

Question

Use the following function rule to find

f(1)

\newline

f(x) = 12(7)^x + 8

\newline

f(1) =

Posted 1 month ago

Question

The town of Valley View conducted a census this year, which showed that it has a population of

1,800

people. Based on the census data, it is estimated that the population of Valley View will grow by

12\%

\newline

Write an exponential equation in the form

y = a(b)^x

that can model the town population,

y

x

decades after this census was taken.

\newline

Use whole numbers, decimals, or simplified fractions for the values of

a

b

\newline

y =

Posted 2 months ago

Question

Solve. Round your answer to the nearest thousandth.

\newline

7 = 9^x

\newline

x =

Posted 2 months ago

Question

Solve. Round your answer to the nearest thousandth.

\newline

7 = e^x

\newline

x =

Posted 1 month ago

Question

Solve. Simplify your answer.

\newline

\log u = 1

\newline

u =

Posted 2 months ago

Question

Solve. Simplify your answer.

\newline

7\log x = 7

\newline

x =

Posted 1 month ago

Question

g(t) = 3^t

change over the interval from

t = 3

t = 4

\newline

\newline

g(t)

3

\newline

g(t)

3

\newline

g(t)

3\%

\newline

g(t)

200\%

Posted 2 months ago

Question

f(x)

6

over every unit interval in

x

f(0) = 0

\newline

Which could be a function rule for

f(x)

\newline

\newline

f(x) = 6^x

\newline

f(x) = 6x

\newline

f(x) = \frac{1}{6^x}

\newline

f(x) = \frac{x}{6}

Posted 2 months ago