Resourcesdown chevron
Testimonials
Plans
Sign in
Sign up
Resourcesright arrow
Testimonials
Plans
Bytelearn - cat image with glassesAI tutor

Welcome to Bytelearn!

Let’s check out your problem:

Simplify to a single trig function with no denominator. csc^(2)theta*cos^(2)theta Answer: theta

Simplify to a single trig function with no denominator.\newlinecsc⁡2θ⋅cos⁡2θ \csc ^{2} \theta \cdot \cos ^{2} \theta \newlineAnswer:

View step-by-step helpView step-by-step help
Homeright chevron icon
Math Problemsright chevron icon
Algebra 2right chevron icon
Product property of logarithmsright chevron icon

Full solution

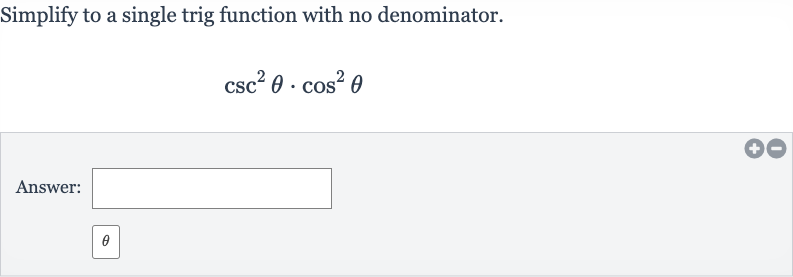
Q. Simplify to a single trig function with no denominator.\newlinecsc⁡2θ⋅cos⁡2θ \csc ^{2} \theta \cdot \cos ^{2} \theta \newlineAnswer:
  1. Recall definition of cosecant function: Recall the definition of the cosecant function. The cosecant function is the reciprocal of the sine function, so csc(θ)=1sin⁡(θ)\text{csc}(\theta) = \frac{1}{\sin(\theta)}.
  2. Rewrite csc⁡2θ\csc^2\theta: Rewrite csc⁡2θ\csc^2\theta as (1/sin⁡(θ))2(1/\sin(\theta))^2. Using the definition from Step 11, we can rewrite csc⁡2θ\csc^2\theta as (1/sin⁡(θ))2(1/\sin(\theta))^2.
  3. Apply power to the fraction: Apply the power to the fraction.\newlineWhen we raise a fraction to a power, we raise both the numerator and the denominator to that power. So, (1/sin⁡(θ))2(1/\sin(\theta))^2 becomes 12/sin⁡2θ1^2/\sin^{2}\theta, which simplifies to 1/sin⁡2θ1/\sin^{2}\theta.
  4. Multiply the expressions: Multiply the expressions.\newlineNow we multiply 1sin⁡2θ\frac{1}{\sin^{2}\theta} by cos⁡2θ\cos^{2}\theta. This gives us (1sin⁡2θ)∗cos⁡2θ\left(\frac{1}{\sin^{2}\theta}\right) * \cos^{2}\theta.
  5. Use Pythagorean identity: Use the Pythagorean identity for sine and cosine.\newlineThe Pythagorean identity states that sin⁡2θ+cos⁡2θ=1\sin^{2}\theta + \cos^{2}\theta = 1. We can rearrange this to express sin⁡2θ\sin^{2}\theta in terms of cos⁡2θ\cos^{2}\theta: sin⁡2θ=1−cos⁡2θ\sin^{2}\theta = 1 - \cos^{2}\theta.
  6. Substitute sin⁡2θ\sin^2\theta: Substitute sin⁡2θ\sin^2\theta in the expression.\newlineSubstitute sin⁡2θ\sin^2\theta with 1−cos⁡2θ1 - \cos^2\theta in the expression from Step 44. This gives us 11−cos⁡2θ×cos⁡2θ\frac{1}{1 - \cos^2\theta} \times \cos^2\theta.
  7. Simplify the expression: Simplify the expression.\newlineSince we have a common factor of cos⁡2θ\cos^{2}\theta in the numerator and the denominator, we can simplify the expression to just cos⁡2θ\cos^{2}\theta. This is because (1/(1−cos⁡2θ))⋅cos⁡2θ=cos⁡2θ/(1−cos⁡2θ)⋅(1−cos⁡2θ)=cos⁡2θ(1/(1 - \cos^{2}\theta)) \cdot \cos^{2}\theta = \cos^{2}\theta / (1 - \cos^{2}\theta) \cdot (1 - \cos^{2}\theta) = \cos^{2}\theta.

More problems from Product property of logarithms

Question
Write the exponential equation in logarithmic form.\newline93=7299^3 = 729\newlinelog⁡□729=3\log_\square 729 = 3
Get tutor helpright-arrow

Posted 2 months ago

Question
Write the exponential equation in logarithmic form.\newlinee4≈54.598e^4 \approx 54.598\newlineln⁡____≈4\ln\_\_\_\_ \approx 4
Get tutor helpright-arrow

Posted 2 months ago

Question
Write the logarithmic equation in exponential form.\newlinelog⁡10100=2\log_{10}100 = 2\newline102=‾10^2 = \underline{\hspace{2em}}
Get tutor helpright-arrow

Posted 2 months ago

Question
Evaluate. Write your answer as a whole number, proper fraction, or improper fraction in simplest form.\newlineln⁡(e)10=\frac{\ln (e)}{10} = ______
Get tutor helpright-arrow

Posted 2 months ago

Question
Rewrite as a quotient of two common logarithms. Write your answer in simplest form.\newlinelog⁡333=\log_3 33 = ______
Get tutor helpright-arrow

Posted 2 months ago

Question
Evaluate. Round your answer to the nearest thousandth.\newlinelog⁡550=\log_{5}50 = ____
Get tutor helpright-arrow

Posted 2 months ago

Question
Which property of logarithms does this equation demonstrate? \newlinelog⁡33+log⁡36=log⁡318\log_3 3 + \log_3 6 = \log_3 18\newlineChoices:\newline(A) Product Property\text{Product Property}\newline(B) Power Property\text{Power Property}\newline(C) Quotient Property\text{Quotient Property}
Get tutor helpright-arrow

Posted 2 months ago

Question
Expand the logarithm. Assume all expressions exist and are well-defined.\newlineWrite your answer as a sum or difference of common logarithms or multiples of common logarithms. The inside of each logarithm must be a distinct constant or variable.\newlinelog⁡(uv)\log(uv)\newline_____
Get tutor helpright-arrow

Posted 2 months ago

Question
Expand the logarithm. Assume all expressions exist and are well-defined.\newlineWrite your answer as a sum or difference of common logarithms or multiples of common logarithms. The inside of each logarithm must be a distinct constant or variable.\newlinelog⁡v7\log v^7 \newline______
Get tutor helpright-arrow

Posted 2 months ago

Question
Expand the logarithm. Assume all expressions exist and are well-defined.\newlineWrite your answer as a sum or difference of base-66 logarithms or multiples of base-66 logarithms. The inside of each logarithm must be a distinct constant or variable.\newlinelog⁡6w6\log_6 w^6\newline______
Get tutor helpright-arrow

Posted 2 months ago

View all (47)