Resourcesdown chevron
Testimonials
Plans
Sign in
Sign up
Resourcesright arrow
Testimonials
Plans
Bytelearn - cat image with glassesAI tutor

Welcome to Bytelearn!

Let’s check out your problem:

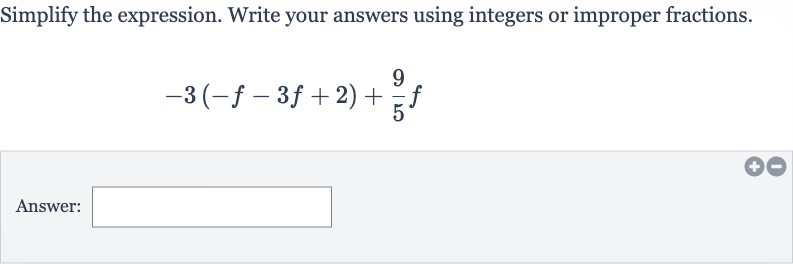
Simplify the expression. Write your answers using integers or improper fractions. -3(-f-3f+2)+(9)/(5)f Answer:

Simplify the expression. Write your answers using integers or improper fractions.\newline−3(−f−3f+2)+95f -3(-f-3 f+2)+\frac{9}{5} f \newlineAnswer:

View step-by-step helpView step-by-step help
Homeright chevron icon
Math Problemsright chevron icon
Algebra 1right chevron icon
Linear equations: solve for yright chevron icon

Full solution

Q. Simplify the expression. Write your answers using integers or improper fractions.\newline−3(−f−3f+2)+95f -3(-f-3 f+2)+\frac{9}{5} f \newlineAnswer:
  1. Distribute Terms: Distribute −3-3 to each term inside the parentheses.\newlineWe have the expression −3(−f−3f+2)-3(-f - 3f + 2). Distribute −3-3 to each term inside the parentheses.\newline−3×−f=3f-3 \times -f = 3f\newline−3×−3f=9f-3 \times -3f = 9f\newline−3×2=−6-3 \times 2 = -6\newlineSo, the expression becomes 3f+9f−63f + 9f - 6.
  2. Combine Like Terms: Combine like terms.\newlineWe have 3f+9f3f + 9f from the previous step. Combine these like terms.\newline3f+9f=12f3f + 9f = 12f\newlineSo, the expression now is 12f−612f - 6.
  3. Add Fraction Part: Add the fraction part of the expression.\newlineWe have the fraction (95)f(\frac{9}{5})f to add to our current expression 12f−612f - 6.\newlineSince 12f12f is the same as (605)f(\frac{60}{5})f, we can write the expression as (605)f−6+(95)f(\frac{60}{5})f - 6 + (\frac{9}{5})f.
  4. Combine Like Terms with Fractions: Combine like terms with fractions.\newlineWe have (605)f+(95)f(\frac{60}{5})f + (\frac{9}{5})f from the previous step. Combine these like terms.\newline(605)f+(95)f=(695)f(\frac{60}{5})f + (\frac{9}{5})f = (\frac{69}{5})f\newlineSo, the expression now is (695)f−6(\frac{69}{5})f - 6.
  5. Final Simplified Expression: The expression is already simplified.\newlineThe final simplified expression is (695)f−6(\frac{69}{5})f - 6, which is in terms of integers and improper fractions.

More problems from Linear equations: solve for y

Question
Does the point (0,4)(0, 4) satisfy the equation y=xy = x??\newlineChoices: [[yes]][[no]]
Get tutor helpright-arrow

Posted 2 months ago

Question
Find the slope of the line y=−4x+25 y = -4x + \frac{2}{5} .\newlineWrite your answer as an integer or as a simplified proper or improper fraction.
Get tutor helpright-arrow

Posted 1 month ago

Question
Rewrite the following equation in slope-intercept form.\newliney+8=−8(x−10)y + 8 = -8(x - 10)\newlineWrite your answer using integers, proper fractions, and improper fractions in simplest form.\newline_____
Get tutor helpright-arrow

Posted 1 month ago

Question
Find the y y -intercept of the line 14x−16y=−10 14x - 16y = -10 .\newlineWrite your answer as an integer or as a simplified proper or improper fraction, not as an ordered pair.\newline____
Get tutor helpright-arrow

Posted 1 month ago

Question
Line q q has a slope of −23 \frac{-2}{3} . Line r r is perpendicular to q q . What is the slope of line r r ?\newlineSimplify your answer and write it as a proper fraction, improper fraction, or integer.\newline_____\_\_\_\_\_
Get tutor helpright-arrow

Posted 1 month ago

Question
Find the slope of the line y+3=−118(x+6)y + 3 = -\frac{1}{18}(x + 6).\newlineWrite your answer as an integer or as a simplified proper or improper fraction.
Get tutor helpright-arrow

Posted 1 month ago

Question
Line p p has an equation of y=−8x+6 y = -8x + 6 . Line q q , which is perpendicular to line p p , includes the point (2,−2) (2, -2) . What is the equation of line q q ??\newlineWrite the equation in slope-intercept form. Write the numbers in the equation as simplified proper fractions, improper fractions, or integers.\newline____
Get tutor helpright-arrow

Posted 1 month ago

Question
Is the graph of this equation a horizontal or vertical line?\newlinex=7x = 7\newlineChoices:\newline(A)horizontal line\text{(A)horizontal line}\newline(B)vertical line\text{(B)vertical line}
Get tutor helpright-arrow

Posted 2 months ago

Question
Rewrite the following equation in standard form.\newliney=−10x+7 y = -10x + 7 \newlineHint: The standard form of a linear equation is\newlineAx+By=C Ax + By = C where A A and B B are not both zero, and A A , B B , and C C are integers whose GCF is 11. ______
Get tutor helpright-arrow

Posted 2 months ago

Question
A line with a slope of 99 passes through the point (9,9)(9,9). What is its equation in point-slope form?\newlineUse the specified point in your equation. Write your answer using integers, proper fractions, and improper fractions. Simplify all fractions.\newliney−‾=‾(x−‾)y - \underline{\hspace{1cm}} = \underline{\hspace{1cm}} (x - \underline{\hspace{1cm}})
Get tutor helpright-arrow

Posted 1 month ago

View all (31)